by Lev Birbrair and Anne Pichon
Anne
I first met Walter in 2002 in New York at a conference on singularity theory he organized with Ágnes Szilárd. He then visited me several times in Marseille, and we wrote two short papers on analytic realizations of multilinks in 3-dimensional manifolds, one with András Némethi. A couple of years later, in June 2009, Walter visited Marseille with his collaborator Lev Birbrair. The first day, they started to explain their seminal results on inner Lipschitz geometry of complex singular germs on the blackboard of the cafeteria, and offered me one of the greatest days — if not the greatest — of my mathematical life. It turned out after a couple of hours that our viewpoints were perfectly complementary and would lead to a global and complete picture of the inner Lipschitz geometry of normal surface germs. At the end of the day, we had a three-line statement that a surface singularity has a natural thick-thin decomposition, similar to the one described by Gregori Margulis for negative curvature spaces. Our collaboration really took off there, and I am very grateful to both of them to have brought me onto the team, and particularly to Walter, who had the idea of this visit in Marseille.
Lev
I learned who Walter Neumann is when I was a student. His classic theorem about plumbing calculus and resolution of complex surface singularities was already famous. We met at several conferences in singularity theory. His talks were always very interesting, but before the Trieste conference in 2005 we never discussed mathematics. His lecture in Trieste stimulated Alexandre Fernandes and me to think about Lipschitz geometry of complex surfaces. When I visited Columbia University that same year, Alexandre and I had the first examples of nonconical singularities of complex surfaces with respect to the inner metric. Walter said to me: “Excellent. The Lipschitz geometry is nonempty. We must see what we can do.” This was the point were the collaboration started. When Anne joined the group, the theory became much deeper. All Walter’s experience and capacity turned out to be extremely useful for the creation of the modern Lipschitz geometry. Walter is a wonderful collaborator and I am very happy that I had the great privilege of working with him.
We will present an overview of the results we proved with Walter on the inner Lipschitz classification of complex surfaces, and then, of some of the results he proved with Anne on their outer Lipschitz geometry and on Lipschitz normal embeddings. We refer to the original papers for precise definitions, statements and proofs, as well as to the recent lecture notes volume on Lipschitz geometry of singularities edited by Walter and Anne [11].
1. Seminal works
Let
How does
look in a neighborhood of the origin?
There are multiple answers to this vague question, depending on the
category we work in. The famous Conical Structure Theorem gives a
complete answer in the topological category: let
How does the link
evolve metrically as tends to zero?
In other words, is
These questions can be approached from two different viewpoints
depending on the choice of the metric. Given an analytic germ
The pioneering work on the Lipschitz geometry of complex spaces is the
1969 paper
[e1]
by
Frédéric Pham
and
Bernard Teissier
(for an
English translation, see the final chapter of
[11]),
where they present a complete classification of the
Lipschitz geometry of complex curves for the outer metric. The higher
dimensions remained unexplored for decades. In particular, the inner
Lipschitz geometry of complex singularities was completely ignored.
Actually, it was known for a long time that complex curve germs are
metrically conical, i.e., inner bi-Lipschitz equivalent to straight
cones over their links, and it was believed by experts that the same
was true in higher dimensions. They were wrong! The first example of a
nonconical complex surface germ was given by Lev and Alexandre
Fernandes in
[e13]:
for
Before presenting Walter’s main contributions to the field, let us say a few words on the reasons that make the Lipschitz geometry of singular spaces so interesting and popular.
First, while the outer and inner metrics on
Another motivation comes from the “tameness” of Lipschitz
classification of space germs. Analytic types of singular complex
space germs contain continuous moduli, and this is why it is difficult
to describe a complete analytic classification. For example, consider
the family of complex plane curves germs
2. The thick-thin decomposition of surfaces and the complete inner Lipschitz classification
When we started to work together with Walter in June 2009 in Marseille, it turned out after a couple of hours that our viewpoints were perfectly complementary. They would eventually lead to a global and complete picture of the inner Lipschitz geometry of normal surface germs. At the end of the day, we had a three-line statement saying that a surface singularity has a natural thick-thin decomposition, similar to the one described by Margulis for negative curvature spaces. From this starting point, it took us more than two years to write a full proof of the theorem and based on it, to give a complete classification of inner geometry of normal complex surface germs, which eventually appeared in [7].
In the first examples of nonconical normal surface germs presented
by Lev, Alexandre Fernandes and Walter, the obstruction to metrical
conicalness of normal is given by the existence of fast loops. A
fast loop in
The key result of
[7]
is the Thick-Thin
Decomposition Theorem (Theorem 1.6 in that article)
which states the
existence and unicity (up to a very natural equivalence relation) of
what we called a (minimal) thick-thin decomposition of every normal
surface germ
As a first application, the thick-thin decomposition enabled us to characterize the normal surface germs which are metrically conical:
The thick-thin decomposition is remarkably related to the topology of
the link
- each
- each
- there
exist rational numbers
One of the remarkable properties of the thick-thin decomposition is
that it can be explicitely described in a very easy way through a
suitable resolution of the singularity by using the plumbing calculus
developed by Walter in
[1]
thirty years before (see
Jonathan Wahl’s contribution in the present volume). Let us describe
this more precisely. Consider the minimal good resolution
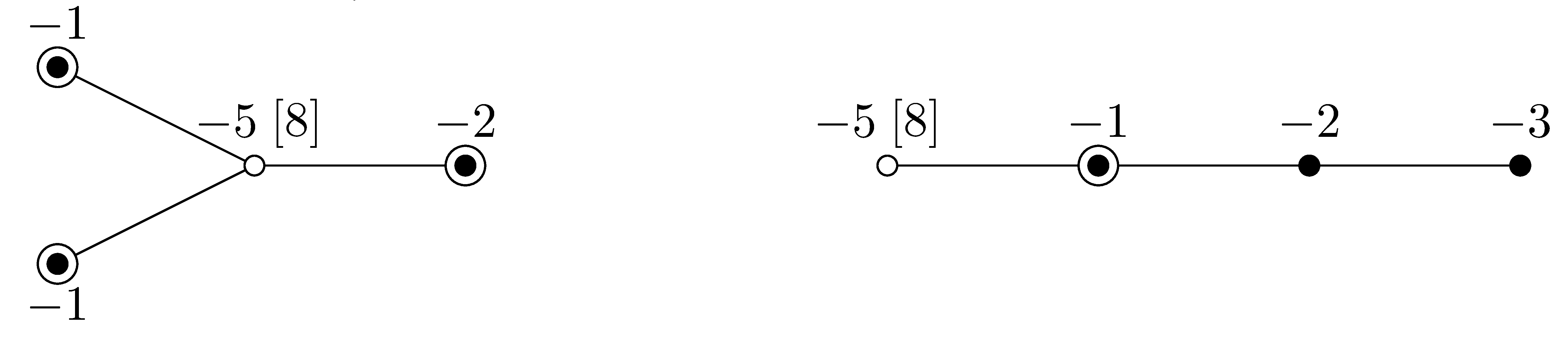
Left:
Many explicit examples are described in
[7].
Let us give just one. Consider the
family of surface singularities Briançon and Speder in
[e2].
It
has constant topological type, while the thick-thin decomposition
changes radically when
The second main result of [7], built on a refinement of the thick-thin decomposition, gives the complete inner Lipschitz classification of normal surface germs:
- the graph decomposition of
- in the notation of Theorem 2.2,
for each thin zone
The higher-dimensional case remains up to now almost unexplored.
3. Outer Lipschitz geometry
As already mentioned, the complete Lipschitz classification of
complex curve germs for the outer metric was established by
Frédéric Pham and Bernard Teissier in
[e1]
using the concept of Lipschitz saturations. In fact, any curve germ
- there is a homeomorphism of germs
- there is a bi-Lipschitz homeomorphism
of germs
The equivalence of (ii) and
(iii) is the result of Pham and
Teissier. The equivalence of (i),
(iii)
and
(iv) is the
new contribution. The proof of
The question of the outer Lipschitz classification of higher-dimensional germs is still open. Actually, a very small amount of analytic invariants are determined by the topological type of an analytic germ (even if one considers the embedded topological type), and a first natural question to ask is whether the Lipschitz classification is sufficiently rigid to catch analytic invariants:
Which analytical invariants are in fact Lipschitz invariants?
During the last decade, it was shown that a large amount of analytic invariants are determined by the outer Lipschitz geometry in the case of a surface germ. The main result of [8] is the pioneering result in this direction. It shows that the outer Lipschitz class contains a lot of information on the singularity and confirms that outer Lipschitz geometry of singularities is a very promising area to explore. It can be seen as a first keystone to a complete classification of the outer Lipschitz geometry of complex surfaces. The higher-dimensional setting remains almost unexplored.
- the decorated resolution graph
of the minimal good resolution of
- the multiplicity of
- the maximal ideal cycle in its resolution;
- for a generic hyperplane
- the decorated resolution
graph of the minimal good resolution of
- the topology of the discriminant curve of a generic plane projection;
- the outer Lipschitz geometry of the polar curve of a generic plane projection.
“Decorated resolution graph” means the resolution graph decorated with arrows corresponding to the components of the strict transform of the resolved curve.
The proof of this result is based on a sophisticated version of the
bubble trick which leads to the graph decomposition of the link
The Lipschitz invariance of the multiplicity (point (ii)
in the above theorem) was then extended
beyond the normal case by Alexandre Fernandes and José Edson Sampaio
([e15])
for a hypersurface in
4. Lipschitz normal embeddings
Another important contribution of Walter in the area of Lipschitz
geometry of singularities is his series of works on Lipschitz normal
embedding. A subset (or a germ) in
The pioneering result on LNE is the following theorem due to Lev and Tadeusz Mostowski. Its original version is proved in the semialgebraic setting, but this result remains true with the same proof in the more general subanalytic category and even, in the o-minimal category. We state it here in the subanalytic setting.
The map
As a consequence of this theorem, one obtains the following alternative definition of the notion of Lipschitz normal embedding. The proof was never written before. That is why we include it in this manuscript.
Proof. Assume there exist a subanalytic bi-Lipschitz homeomorphism
Complex and real algebraic sets of
The answer is positive in complex dimension 1: every complex
algebraic curve admits a complex algebraic Lipschitz normal embedding.
This follows from the fact that an irreducible germ of complex
algebraic curve is subanalytically bi-Lipschitz homeomorphic with
respect to the inner metric to the germ of
Walter, Lev and Alexandre Fernandes answered negatively to Mostowski’s question in higher dimension by proving the following result:
The proof of this result is based on a beautiful topological argument due to Walter that we reproduce here:
Proof. Assume there exists a complex algebraic Lipschitz normal embedding
Another important contribution of Walter is a characterization of
normal surface singularities which are LNE. In
[e16],
Lev proved with
Rodrigo Mendes
that a
semialgebraic germ
This efficient LNE criterion led to the discovery of several nontrivial infinite family of LNE normal surface germs. For example:
Minimal singularities were introduced by Janos Kollár in [e5]. In dimension 2, they are rational surface singularities which play a major role in resolution theory (see [e7], [e10]).
Several other families were discovered later using the criterion of
[9],
for example among super-isolated
singularities
[e21].
But LNE-ness of complex surfaces
is far from being completely understood, and the higher-dimensional
case is still in its infancy.
5. Dissemination
In June 2018, Walter organized with Anne an international school on Lipschitz geometry of singularities in Cuernavaca, Mexico. About fifty PhD students and young researchers attended the event, and the theory and promising extensions were introduced a huge variety of viewpoints, by Walter himself and also Patrick Popescu-Pampu, Maria Aparecida Soares Ruas, Bernard Teissier, David Trotman, among others.
The lecture notes were completed and collected in a Lecture Notes in Mathematics volume [11], edited by Walter and Anne, which is the first introductory book on Lipschitz geometry of singularities, and which is now in the hands of many PhD students and young researchers. The volume also contains an english version of the historical paper of Pham and Teissier [e1], which was a preprint in French of the École Polytechnique during 50 years.