Celebratio Mathematica

In re­cent years it has be­come de ri­gueur for an in­vited speak­er at a con­fer­ence to “Thank the or­gan­izers for in­vit­ing me.” Today I can say this with more than the usu­al sin­cer­ity. Par­is is my fa­vor­ite city in the world, I have many fond memor­ies of the In­sti­tut Henri Poin­caré, and the sub­ject of this con­fer­ence is very close to my heart. So to Claude, and all the or­gan­izers who have worked hard to make this con­fer­ence a suc­cess. Merci bi­en.

Today I would like to tell you the story of per­haps the most en­joy­able and stim­u­lat­ing col­lab­or­a­tion of my ca­reer — my in­ter­ac­tion with Luc Bénard. Luc is a tal­en­ted cre­at­or of breath­tak­ing math­em­at­ic­al art, and it really should be Luc stand­ing here giv­ing a talk at this con­fer­ence, but un­for­tu­nately oth­er com­mit­ments pre­ven­ted him from com­ing to Par­is at this time.

While I ap­pre­ci­ate math­em­at­ic­al art, I have little tal­ent for cre­at­ing it, and what I brought to our part­ner­ship was primar­ily tech­nic­al know­ledge and ex­per­i­ence in cre­at­ing soft­ware tools for rep­res­ent­ing math­em­at­ic­al ob­jects as com­puter-based im­ages. True, Luc has made good use of these tools, but I feel some­what em­bar­rassed by the ex­cess cred­it I have re­ceived for his cre­ations; it is as if one gave par­tial cred­it for the Mona Lisa to the ar­tis­an who cre­ated da Vinci’s paint­brushes.

I looked back at my early email mes­saging with Luc re­cently. It shows that in early Decem­ber of 2004, Luc was us­ing my soft­ware, 3D-XplorMath (3DXM) and asked if it would be pos­sible to save sur­faces cre­ated with the pro­gram in a cer­tain stand­ard format (.obj), since that would make it pos­sible for him to use oth­er 3D graph­ics pro­grams he liked a lot (Bryce and Car­rara) to fur­ther pro­cess these sur­faces. Since the Aus­trali­an as­tro­phys­i­cist and com­puter sci­ent­ist, Paul Bourke, was an ex­pert in such mat­ters (as well as hav­ing one of the best Math­em­at­ic­al Visu­al­iz­a­tion web­sites on the Net) we asked him for help, and over the next few weeks Paul and I im­ple­men­ted sav­ing 3DXM gen­er­ated sur­faces as .obj files with Luc as our beta-test­er.

The pay­off came in the form of a spec­tac­u­lar New Year’s Day present from Luc!

From: Luc Benard ludev@video­tron.ca
Date: Janu­ary 1, 2005 10:26:07 AM EST (CA)
Sub­ject: Re: surf format
Happy New Year to you and your fam­ily,

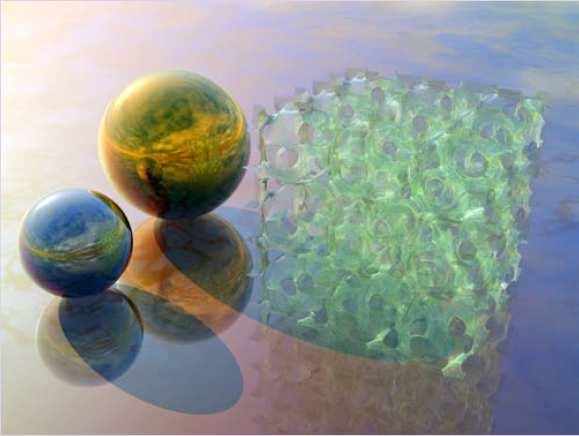
I couldn’t be­lieve my eyes! The il­lu­sion of real­ity was so strong that I was com­pletely con­vinced by it. I im­me­di­ately re­cog­nized the sur­faces as hav­ing ori­gin­ated from my own pro­gram, 3D-XplorMath, but I nev­er­the­less be­lieved that this had to be a pho­to­graph of real, phys­ic­al glass mod­els!

And I wasn’t the only one fooled by it. Here is a story I told to many people as I showed them Luc’s im­age — and most of them ac­cep­ted it: These sur­faces were ini­tially cre­ated in soft­ware, us­ing the math­em­at­ic­al visu­al­iz­a­tion pro­gram called 3D-XplorMath. The res­ult­ing im­ages were giv­en to a highly skilled ar­tis­an glass-blower from Mur­ano, who fab­ric­ated them out of thin colored glass. The glass ob­jects were then giv­en to a pro­fes­sion­al pho­to­graph­er who placed them art­fully on a glass covered wal­nut tab­letop and took their pic­ture.

Of course, the vir­tu­al glass blower and vir­tu­al pho­to­graph­er were both Luc Bénard, from Montreal, not Mur­ano. Here is my reply mes­sage to Luc. Note that I copied to many of my friends who are in­ter­ested in math­em­at­ic­al visu­al­iz­a­tion.

To:Luc Benard <ludev@video­tron.ca>
Date: Janu­ary 1, 2005 4:29:23 PM EST (CA)
Cc:Paul Bourke <paul.bourke@gmail.com>, dave hoff­man <dav­id@msri.org> karch­er
<karch­er@math.uni-bonn.de>, xah lee <xah@xah­lee.org>, <mat­weber@in­di­ana.edu>,
Mar­tin Guest <mar­tin@comp.metro-u.ac.jp>, eck@hws.edu

Dear Luc,
When I looked at your at­tach­ment I could hardly be­lieve my eyes! My first im­pres­sion was that this must surely be a care­ful high res­ol­u­tion di­git­al pho­to­graph of real glass mod­els sit­ting on a real glass tab­letop and made by some in­cred­ibly skilled glass­blower…

One of the people I sent Luc’s pic­ture to was my daugh­ter, Ju­lie Pal­ais, who is a pro­gram dir­ect­or for the US Na­tion­al Sci­ence Found­a­tion. She liked the pic­ture a lot and sug­ges­ted that we sub­mit it to the Sci­ence and En­gin­eer­ing Visu­al­iz­a­tion Chal­lenge, that each year is sponsored jointly by the NSF and Sci­ence Magazine. Luc and I de­cided to do that, al­though we felt we would be lucky to even get an hon­or­able men­tion; Sci­ence Magazine is not very math ori­ented, tend­ing to fa­vour the “hard” sci­ences.

BUT, much to our sur­prise, we won First Prize in the 2006 com­pet­i­tion, and Luc’s “Five Glass Sur­faces on a Table Top” be­came the cov­er il­lus­tra­tion of the Sept. 22, 2006 is­sue of Sci­ence.

I will come back to the “Five Sur­faces” later and show you a sample of all the very hard work that Luc put in­to pro­du­cing the fi­nal im­age. But first let me tell you about an­oth­er beau­ti­ful sur­face im­age that Luc cre­ated about a year ago. This one is of a single sur­face, the pseudo­spher­ic­al Kuen’s Sur­face, and it is in­ten­ded to be more than just a pretty pic­ture — as I will ex­plain, it tells the story of a highly com­plex his­tor­ic­al de­vel­op­ment that stretches from Eu­c­lid’s Ax­ioms to mod­ern Quantum Field The­ory, and we de­cided to enter it in­to the 2009 Sci­ence and En­gin­eer­ing Visu­al­iz­a­tion Com­pet­i­tion.

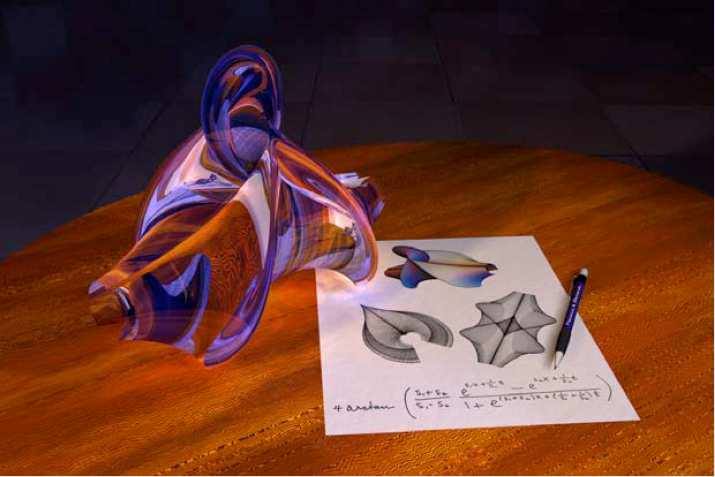
For 2000 years math­em­aticians searched for a proof that Eu­c­lid’s oth­er pos­tu­lates lo­gic­ally im­plied his Fifth or Par­al­lel Pos­tu­late: “Through a point out­side a giv­en line there can be drawn ex­actly one par­al­lel line”. Fi­nally, in 1826, Nikolai Lob­achevsky showed that this was a fu­tile goal; he con­struc­ted a geo­metry that sat­is­fied the oth­er Eu­c­lidean pos­tu­lates, but not the Fifth. In­deed, in this new geo­metry there were in­fin­itely many par­al­lel lines through the giv­en point. Such geo­met­ries are now some­times called “Lob­achevski­an”, but more com­monly are called “hy­per­bol­ic”, and sur­faces in space that ex­hib­it this geo­metry are called “pseudo­spher­ic­al”, after the simplest ex­ample, the Pseudo­sphere (which looks like two dunce caps held brim to brim). But just as in ad­di­tion to the plane it­self there are many “planar” sur­faces in space, such as cones and cyl­in­ders, that obey Eu­c­lid’s ax­ioms for plane geo­metry, so too there are many pseudo­spher­ic­al sur­faces be­sides the Pseudo­sphere. A fam­ous ex­ample, is Kuen’s Sur­face, that has been ad­mired for its grace­ful beauty since its dis­cov­ery 150 years ago.

One of their re­mark­able dis­cov­er­ies was that pseudo­spher­ic­al sur­faces were in one-to-one cor­res­pond­ence with the solu­tions of a cer­tain non­lin­ear par­tial dif­fer­en­tial equa­tion that we now call the Sine-Gor­don Equa­tion. Usu­ally such equa­tions do not have ex­pli­cit solu­tions, but Bäcklund dis­covered an im­port­ant se­quence of so- called soliton solu­tions that are ex­pli­cit and which moreover cor­res­pond to par­tic­u­larly beau­ti­ful and sym­met­ric pseudo­spher­ic­al sur­faces. In fact, the Pseudo­sphere is a one-soliton sur­face and Kuen’s Sur­face is a two-soliton. A cen­tury later this same Sine-Gor­don Equa­tion mys­ter­i­ously re­sur­faced in an en­tirely dif­fer­ent con­text: it turned out to be a mod­el of a Re­lativ­ist­ic Quantum Field The­ory, the kind of math­em­at­ic­al struc­ture on which the­or­et­ic­al phys­i­cists base their most ad­vanced and soph­ist­ic­ated the­or­ies of the struc­ture of mat­ter.

Our visu­al­iz­a­tion cap­tures some of the sur­pris­ing mys­tery of this two-thou­sand year saga of in­tim­ately in­ter­con­nec­ted math­em­at­ic­al ideas. Our eyes per­haps dwell first on the planar ob­jects: the floor with its two sets of par­al­lel lines, the table-top and the piece of pa­per with its planar sketches of the Kuen Sur­face seen from vari­ous dir­ec­tions and an eso­ter­ic for­mula writ­ten be­low them. But though that for­mula lies in a plane, it is in fact the two-soliton solu­tion of the Sine-Gor­don Equa­tion, and so de­scribes the glassy Kuen Sur­face ly­ing next to it, in whose pseudo­spher­ic­al shape we can see re­flec­ted the planar table top and scrap of pa­per with its im­ages and its for­mula.

For­tune smiled on us again; the judges liked our visu­al­iz­a­tion and the story be­hind it, and they awar­ded us an­oth­er First Prize for the 2009 com­pet­i­tion. Luc Bénard has pro­duced a great many math­em­at­ic­al visu­al­iz­a­tions of vari­ous types, and I asked him re­cently which ones he liked the best. Let me show you a few of his fa­vor­ites — ac­com­pan­ied by some of his artist­ic philo­sophy in his own words. Then, in what time re­mains, I will try to ex­plain a little bit about Luc’s meth­ods for cre­at­ing these won­der­ful im­ages.

Be­cause I love math­em­at­ic and I al­ways find math­em­at­ic­al curves and sur­face grace­ful and really close to the curves and sur­faces we find in the or­gan­ic world I tried to use them as ob­jects in 3D im­ages sim­u­lat­ing real­ity. Here is an ex­ample, The Triply Peri­od­ic Sur­face WP.

An­oth­er ex­ample is the Fractal Basin: take four highly re­flect­ive tan­gent spheres with equal radii and centres at the ver­tices of a tet­ra­hed­ron. You can use 3D soft­ware to rep­lic­ate this real­ity, in­clud­ing some of it’s phys­ic­al char­ac­ter­ist­ics, in­clud­ing the re­flec­tion of light. if you look in­to the space between the sphere you see a fractal. Here it is with two zooms.

Here is an­oth­er one of Luc’s sur­face im­ages — one of my fa­vor­ites. It shows two glassy Cyc­lides side by side, each re­flect­ing the oth­er.

This is a fant­ast­ic fractal!

This is an­oth­er one of my fa­vor­ites…