by J. Elisenda (Eli) Grigsby
When I first met Rob, I was a first-year grad student at Berkeley, and he was teaching Berkeley’s second-semester algebraic topology course. My dominant memories of Rob from that time were: (i) he rarely wrote anything on the board, and (ii) he seemed genuinely taken aback whenever I called him Professor Kirby. I quickly stopped.
The course itself was a turning point for me. I credit his Poincaré homology sphere homework problem (picture below) for hooking me on low-dimensional topology. It was such a cool mix of a lot of different ideas, and it was fun to hear the history: that Poincaré’s original conjecture was about homology, not homotopy, and Poincaré had discovered this counterexample to his own original conjecture, which led him to revise it.
I was taking a commutative algebra class at the same time. Those problem sets felt like work, but Rob’s didn’t. I now see that this was partly about the math and partly about Rob, and that these two facts are connected. Math is created in communities, and mathematical communities have a tone to them which is set early on and difficult-to-budge later.
When it was time to pick an advisor, I knew it would be Rob. Some older students warned me he was very hands-off and didn’t give thesis problems, but his style turned out to be just right for me. He was always ready to talk math; he asked questions, and was interested in what I had to say — unless it wasn’t interesting, in which case he’d gently tell me so. He was supportive in an authentic, math-centered way. This helped me develop my own compass.
During my second year, he gave a topics course on Heegaard Floer homology. This was less than a year after Peter Ozsváth and Zoltán Szabó posted their first two foundational papers, [e2], [e1]. Thanks to Rob, we all got a head start learning a theory that now, 20 years later, has become required background for many 3.5-dimensional topologists.
Back then, he and Paul Melvin were also working on finding a purely combinatorial description of the theory (as were many others, I’d bet), and he was very open about discussing their strategy. Eventually I started thinking about the problem too. Other advisors might have become guarded at this point, or discouraged me, or warned me off of the problem. But this wasn’t Rob’s style. He was happy to have another person involved, and some of my fondest and most formative mathematical moments were those spent discussing holomorphic structures on branched covers of the disk with Rob and Paul in Banff (2003), then Gokova and Budapest (2004).
Like all grad students, I struggled. A lot. Rob’s response was always something along the lines of: “Don’t work so hard. You have to make sure you’re enjoying the math you’re doing. Otherwise, what’s the point?” This was — of course — incredibly irritating to hear at the time. Sure. Easy for you to say. You’re a professor at Berkeley. But of course he was right. Math is a marathon, not a sprint. Enjoying the scenery you’re helping to paint is the whole point.
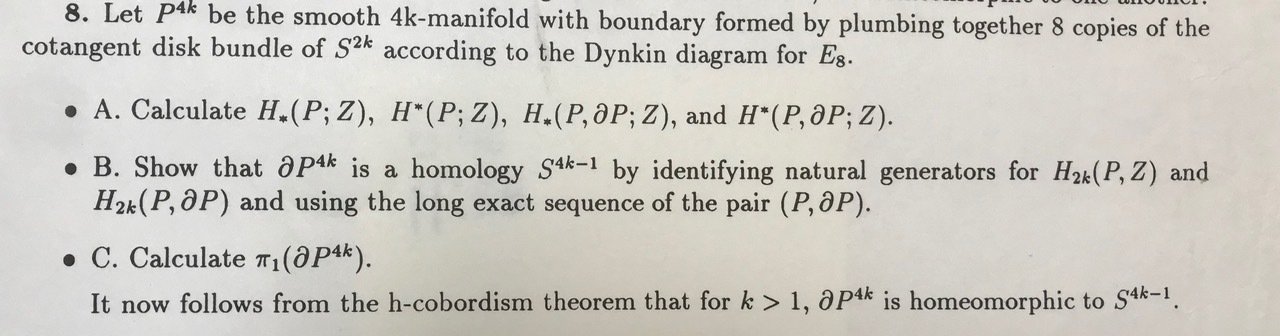
This is all to say that there is a lightness and ease with which Rob moves through mathematics and life, and I have observed this same grace throughout his circle. It may be hyperbolic (pun intended) of me to say, but I’ll go ahead: I think this grace has touched the whole field of low-dimensional topology. I have personally found this community to be a little bit friendlier than others I’ve inhabited. People are just a bit more likely to credit and support each other; to ask questions because they really want to know the answer, and not because they want to show how much they already know.
Five years ago, I mentioned this to my then-partner, who was struggling with setting the right tone at his start-up company, and he wrote to Rob for advice. Rob’s response:
I think I was lucky to start out […] with a good bunch of students […], and they often passed on traditions to the younger students.
Also, I occasionally passed on the comment that it was always a good idea to be extra gracious in referring to other people’s work, or including someone as a coauthor; that those notions paid off handsomely in a nicer community to work in. My father used to talk about upward spirals, in which you normally react to another person in a slightly (epsilon) nicer way than you might be inclined to, and then the response may be slightly nicer and an upward spiral is started. Of course epsilon has to be small, or you become a phony.
This is Rob all over. Without any fanfare, he has quietly nudged us all upward.