Celebratio Mathematica

Dear Yves,

How are you? Thi­erry told me that you’d been very ill, but I hope you’re fully re­covered?

The con­fer­ence in Mar­seille was very in­ter­est­ing. I met there, among oth­ers, Paul Fed­er­bush, an Amer­ic­an phys­i­cist from Ann Ar­bor (Michigan), who was in­ter­ested in your basis. For the ap­plic­a­tions he has in mind, he wanted a basis con­struc­ted also from dilata­tions and trans­la­tions, but one that would stop at a cer­tain scale, in­stead of us­ing ever big­ger cubes. Some­what like the Haar basis: in­stead of tak­ing

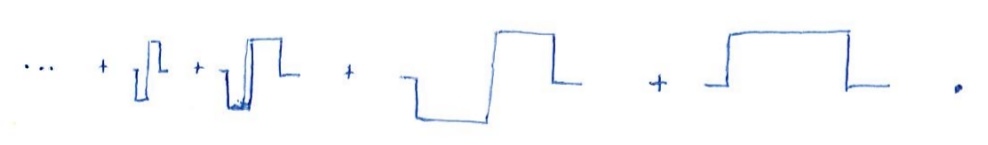
Fed­er­bush’s ques­tion was then wheth­er the same thing is pos­sible with your basis. And the an­swer of course is yes. Ob­vi­ously I’m not telling you any­thing new: it’s all there in your con­struc­tion, im­pli­citly.

It suf­fices to take (in $n$ di­men­sions) $$\begin{array}{rl}\mathcal{B}=\{{\psi }_Q^{(\epsilon )};& {\epsilon }_j=\text{0 or 1},({\epsilon }_1,\dots ,{\epsilon }_n)\ne (0_1,\dots ,0),\\ & Q=[2^{-j}\underset{\sim }{k},2^{-j}(\underset{\sim }{k}+\underset{\sim }{1})[,\underset{\sim }{k}\in {\mathbb{Z}}^n,\underset{\sim }{?}=(1,\dots ,1),\\ & j\in \mathbb{Z}\text{ and }\underset{―}{j\ge j_0}\}\\ & \searrow \\ & {}_{\text{We stop at step }{j}_0;\text{ the largest}}\\ & {}_{\text{cubes considered to}}\\ & {}_{\text{have a side of length }{2}^{-j_0}.}\\ \cup & \{{\psi }_{j_0,\underset{\sim }{k}}^{0,\dots ,0)};\underset{\sim }{k}\in {\mathbb{Z}}^n\}\end{array}$$ where $${\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}(x)=2^{n{j}_{0/2}}\prod _{m=1}^n\phi (2^{j_0}{x}_m)$$ (with the same nota­tion as in your art­icle with P. G. Lemarié). It is easy to see that $$({\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)},{\psi }_{j_0,{\underset{\sim }{k}}^{\prime }}^{(0,\dots ,0)})={\delta }_{\underset{\sim }{k},{\underset{\sim }{k}}^{\prime }}(\ast )$$ (es­sen­tially be­cause $\sum _{l\in \mathbb{Z}}|\hat{\phi }(t+2\pi l){|}^2=1$). If I set $$\begin{array}{rl}{D}_j^{(\epsilon )}& =\sum _{\underset{\sim }{k}\in {\mathbb{Z}}^n}⟨\cdot ,{\psi }_{Q_{j,\underset{\sim }{k}}}^{(\epsilon )}⟩{\psi }_{Q_{j,\underset{\sim }{k}}}^{(\epsilon )}\\ & (\text{where }{Q}_{j,\underset{\sim }{k}}=[2^{-j}\underset{\sim }{k},2^{-j}(\underset{\sim }{k}+\underset{\sim }{1})[=\prod _{m=1}^n[2^{-j}{k}_m,2^{-j}(k_m+1)[)\\ D_j& =\sum _{(\epsilon )\ne (0,\dots ,0)}{D}_j^{(\epsilon )}\\ \text{ and }{E}_j& =\sum _{\underset{\sim }{k}\in {\mathbb{Z}}^n}⟨\cdot ,{\psi }_{j,\underset{\sim }{k}}^{(0,\dots 0)}⟩{\psi }_{j,\underset{\sim }{k}}^{(0,\dots 0)}\end{array}$$ then we have (as you showed me), $$D_j=E_{j+1}-E_j\text{(even in }n\text{ dimensions).}$$ It fol­lows that $$\sum _{j=j_0}^{\mathrm{\infty }}{D}_j=\text{𝟙}-E_{j_0}\text{(in the strong limit sense)},$$ and so $$(\sum _{j=j_0}^{\mathrm{\infty }}{D}_j){\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}=0$$ be­cause $E_{j_0}{\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}={\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}$ by ($\ast$). It fol­lows that

1. $⟨{\psi }_{j,\underset{\sim }{k}}^{(\epsilon )},{\psi }_{j_0,{\underset{\sim }{k}}^{\prime }}^{(0,\dots ,0)}⟩=0$ for every $(\epsilon )\ne (0,\dots ,0)$, every $j\ge j_0$ and every $k,k^{\prime }\in {\mathbb{Z}}^n$;
2. the set of vec­tors $\mathcal{B}$ is there­fore an or­thonor­mal set;
3. the set of vec­tors $\mathcal{B}$ is an or­thonor­mal basis (since $\sum _{j=j_0}^{\mathrm{\infty }}{D}_j+E_{j_0}=\text{𝟙}$).

Paul Fed­er­bush as­sured me that all that is very use­ful to him for us­ing in con­struct­ive field the­ory (I my­self don’t know any­thing about that).

Could you please send me your most re­cent pa­pers about wave­lets? I will be in Brus­sels in Au­gust, in Mar­seille in Septem­ber and in New York from Oc­to­ber on.

With all my friend­ship,

In­grid

$$\star \star \star$$

Cher Yves,

Com­ment vas-tu? Thi­erry m’a ra­conté que tu avais été très mal­ade; j’espère que tu es com­plètement re­mis?

La conférence à Mar­seille était très intéress­ante. J’y ai re­con­tré, en­tr’autres, un phys­i­cien améri­cain, du nom de Paul Fed­er­bush, d’Ann Ar­bor (Michigan), qui était fort intéressé par ta base. Pour les ap­plic­a­tions dont il a be­soin, il préférait une base qui se con­tru­isait aus­si par dilata­tions et trans­la­tions, mais qui s’arrêterait à une cer­taine échelle, au lieu d’util­iser des cubes tou­jours plus grands. Un peu comme dans la base de Haar: au lieu de pren­dre

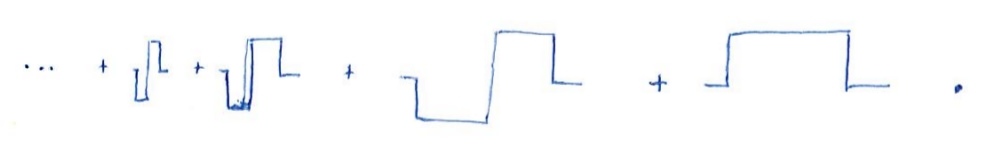
La ques­tion de Fed­er­bush était donc de sa­voir si la même chose était pos­sible avec ta base. Et la réponse est oui, évidem­ment. Je ne t´ap­prends sans doute ri­en de nou­veau: tout se trouve déjà im­pli­cite­ment dans ta con­struc­tion.

Il suf­fit de pren­dre (en $n$ di­men­sions) $$\begin{array}{rl}\mathcal{B}=\{{\psi }_Q^{(\epsilon )};{\epsilon }_j& =\text{0 or 1},({\epsilon }_1,\dots ,{\epsilon }_n)\ne (0_1,\dots ,0),\\ Q& =[2^{-j}\underset{\sim }{k},2^{-j}(\underset{\sim }{k}+\underset{\sim }{1})[,\underset{\sim }{k}\in {\mathbb{Z}}^n,\underset{\sim }{?}=(1,\dots ,1),\\ j& \in \mathbb{Z}\text{et}\underset{―}{j\ge j_0}\}\\ & \searrow \\ & {}_{\text{On s’arrête à l’échelle }{j}_0;\text{ les plus}}\\ & {}_{\text{gros cubes considérés ont}}\\ & {}_{\text{un côte de largeur }{2}^{-j_0}.}\\ & \cup \{{\psi }_{j_0,\underset{\sim }{k}}^{0,\dots ,0)};\underset{\sim }{k}\in {\mathbb{Z}}^n\}\end{array}$$ où $${\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}(x)=2^{n{j}_{0/2}}\prod _{m=1}^n\phi (2^{j_0}{x}_m)$$ avec les mêmes nota­tions que dans ton art­icle avec P. G. Lemarié). Il est fa­cile de voir que $$({\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)},{\psi }_{j_0,{\underset{\sim }{k}}^{\prime }}^{(0,\dots ,0)})={\delta }_{\underset{\sim }{k},{\underset{\sim }{k}}^{\prime }}(\ast )$$ (es­sen­ti­elle­ment parce que $\sum _{l\in \mathbb{Z}}|\hat{\phi }(t+2\pi l){|}^2=1$). Si je dénote $$\begin{array}{rl}{D}_j^{(\epsilon )}& =\sum _{\underset{\sim }{k}\in {\mathbb{Z}}^n}⟨\cdot ,{\psi }_{Q_{j,\underset{\sim }{k}}}^{(\epsilon )}⟩{\psi }_{Q_{j,\underset{\sim }{k}}}^{(\epsilon )}\\ & (\text{où }{Q}_{j,\underset{\sim }{k}}=[2^{-j}\underset{\sim }{k},2^{-j}(\underset{\sim }{k}+\underset{\sim }{1})[=\prod _{m=1}^n[2^{-j}{k}_m,2^{-j}(k_m+1)[)\\ D_j& =\sum _{(\epsilon )\ne (0,\dots ,0)}{D}_j^{(\epsilon )}\\ \text{ et }{E}_j& =\sum _{\underset{\sim }{k}\in {\mathbb{Z}}^n}⟨\cdot ,{\psi }_{j,\underset{\sim }{k}}^{(0,\dots 0)}⟩{\psi }_{j,\underset{\sim }{k}}^{(0,\dots 0)}\end{array}$$ al­ors (comme tu l’as mon­tré), $$D_j=E_{j+1}-E_j\text{(même en }n\text{ dimensions).}$$ Il s’en­suit que $$\sum _{j=j_0}^{\mathrm{\infty }}{D}_j=\text{𝟙}-E_{j_0}\text{(au sense de la limite forte)},$$ et donc $$(\sum _{j=j_0}^{\mathrm{\infty }}{D}_j){\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}=0$$ puisque $E_{j_0}{\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}={\psi }_{j_0,\underset{\sim }{k}}^{(0,\dots ,0)}$ par ($\ast$). Il en res­ulte que

1. $⟨{\psi }_{j,\underset{\sim }{k}}^{(\epsilon )},{\psi }_{j_0,{\underset{\sim }{k}}^{\prime }}^{(0,\dots ,0)}⟩=0$ pour tout $(\epsilon )\ne (0,\dots ,0)$, pour tout $j\ge j_0$ pour tout $k,k^{\prime }\in {\mathbb{Z}}^n$;
2. l’en­semble des vec­teurs $\mathcal{B}$ est donc un en­semble or­thonor­mal;
3. l’en­semble des vec­teurs $\mathcal{B}$ est une base or­thonor­male (puisque $\sum _{j=j_0}^{\mathrm{\infty }}{D}_j+E_{j_0}=\text{𝟙}$).

Paul Fed­er­bush m’a as­suré que tout ceci lui était ex­trêmement utile pour la théorie con­struct­ive des champs (dont je ne con­nais ri­en moi-même).

Pour­rais-tu, s’il-te-plaît, m’en­voy­er tes derniers papi­ers sur les ondelettes? Je serai à Bruxelles en août, à Mar­seille en septembre, et à New York à partir d’oc­tobre.

Avec toutes mes amitiés,

In­grid