Celebratio Mathematica

Dear Yves,

How are you? I’ve been mean­ing to write for a long time, and I’m a bit em­bar­rassed that I’ve waited so long. But here fi­nally are some news.

Ann Ar­bor has offered us, Robert and me both, as­so­ci­ate pro­fess­or­ships (“with ten­ure”). They told me that they were quite im­pressed by your let­ter of re­com­mend­a­tion, for which I would like to thank you again. Moreover, Bell Labor­at­or­ies has also made me an of­fer (Robert is work­ing there already). We have to de­cide be­fore March 1 (one week to go…) If it were only up to me, I think I’d likely go for Ann Ar­bor: I like the area very much, and it is a good uni­versity. And I would learn a lot of math there. On the oth­er hand, Robert is rather more at­tached to Bell Labs than I thought (and than he him­self thought, in fact), and, since I don’t want to start our mar­riage by tak­ing him away from Bell when he likes it there, we will prob­ably opt for the Bell al­tern­at­ive. Ac­tu­ally I think I’ll en­joy Bell too, quite a bit: there are very good math­em­aticians there too, and they give their re­search­ers a lot of free­dom. You’d said that, if we went to Ann Ar­bor, you’d come vis­it us — Robert and me and the kids. I hope you will come see us even if we cast our lot with Bell! I think you will find in­ter­est­ing cer­tain of the math­em­aticians (An­drew Odlyzko, Ron Gra­ham, Larry Shepp, Jeff Lagari­as, Neil Sloane,⁠…). They too were very im­pressed by your re­com­mend­a­tion. Thanks for open­ing those doors to me!

While wait­ing to de­cide with fi­nal cer­tainty (which will hap­pen this week), I have fi­nally fin­ished the big pa­per on frames (which I was really fed up with by the end), and re­turned to wave­lets. The ar­rival of Stéphane Mal­lat’s pa­per, which I thought very beau­ti­ful, led me to mulling on or­thonor­mal wave­let bases. I wondered, too, wheth­er a “pyr­am­id” al­gorithm such as the one in Mal­lat’s pa­per must ne­ces­sar­ily be foun­ded on a wave­let basis. I dis­cussed this with the “vis­ion” people here, and they seem to think that an or­tho­gon­al­ity prop­erty for sub­spaces of ${\ell }^2(\mathbb{Z})$, which then re­quires the ex­ist­ence of an or­thonor­mal wave­let basis un­der­ly­ing the “pyr­am­id”, is es­sen­tial. This whole thing has led me also to the con­struc­tion of or­thonor­mal bases of wave­lets with com­pact sup­port. (Here am I, who had al­ways worked with frames, sur­ren­der­ing to or­thonor­mal­ity too!) Such bases ex­ist, but the ones I’ve found look a bit strange.

The idea is the fol­low­ing.

Let’s try to con­struct se­quences $f(n),g(n)$ such that $$\begin{array}{}\text{(1)}& \sum _{k}f(n-2k)f^{\ast }(\ell -2k)+g(n-2k)g^{\ast }(\ell -2k)={\delta }_{n\ell }.\end{array}$$ (In es­sence, that’s all that’s needed for an ${\ell }^2(\mathbb{Z})$ de­com­pos­i­tion-and-re­con­struc­tion “pyr­am­id” to work.)

We can then define $G,F:{\ell }^2(\mathbb{Z})\to {\ell }^2(\mathbb{Z})$ by $$(Fc{)}_k=\sum _{n}f(n-2k)c_n,(Gc{)}_k=\sum _{n}g(n-2k)c_n.$$ Then $F^{\ast }F+G^{\ast }G=\text{𝟙}$, and we have $$(F^{\ast }{)}^N{F}^N+\sum _{j=1}^N{F}^{\ast N-j}{G}^{\ast }G{F}^{N-j}=\text{𝟙},$$ that is, a de­com­pos­i­tion-and-re­con­struc­tion of ${\ell }^2(\mathbb{Z})$ with a pyr­am­id al­gorithm. In prac­tice, we will choose $f,g$ to be real.

It seems reas­on­able (but not ne­ces­sary) to lim­it ourselves to the case where $$F^{\ast }{\ell }^2(\mathbb{Z})\perp G^{\ast }{\ell }^2(\mathbb{Z})$$ (it is clear that the sum of the two spaces is ${\ell }^2$ in all cases). This would lead (ac­cord­ing to the in­tu­ition of the vis­ion people) to a great­er de­gree of data com­pac­tion than the non-or­tho­gon­al case. So we im­pose the con­di­tion $$\begin{array}{}\text{(2)}& \sum _{n}f(n-2k)g^{\ast }(n-2\ell )=0.\end{array}$$ Clearly all the con­di­tions are sat­is­fied if the $f,g$ are ob­tained start­ing from a wave­let basis. On the oth­er hand, if $$\begin{array}{}\text{(3)}& \sum _{n}f(n)=\sqrt{2},\sum _{n}g(n)=0\end{array}$$ (these two con­di­tions are equi­val­ent if all the oth­ers hold), and if $$F(\xi )=\frac{1}{\sqrt{2}}\sum _{n}f(n)e^{in\xi }$$ sat­is­fies $$\prod _{j=1}^{\mathrm{\infty }}F(2^{-j}\xi )\in L^2(\mathbb{R}),$$ then we have an as­so­ci­ated wave­let basis: $$\begin{array}{c}\hat{\phi }(\xi )=\prod _{j=1}^{\mathrm{\infty }}F(2^{-j}\xi ),\psi (x)=\sum _{k}g(k)\sqrt{2}\phi (2x-k),\\ ⟨{\phi }_{-1k},{\phi }_{0n}⟩=f(n-2k),\\ ⟨{\psi }_{-1k},{\phi }_{0n}⟩=g(n-2k).\end{array}$$ If we in­sist, at the start, that the se­quences $f(n),g(n)$ only have fi­nitely many nonzero terms, the as­so­ci­ated $\psi ,\phi$ will have com­pact sup­port (in that case $F$, and there­fore also $\prod _{j=1}^{\mathrm{\infty }}F(2^{-j}\xi )$, are en­tire and of ex­po­nen­tial type).

So the ques­tion is to find $f,g$ sat­is­fy­ing all the con­di­tions.

If we set $$\begin{array}{rlrl}f(2n)& =a(n),& g(2n)& =c(n),\\ f(2n+1)& =b(n),& g(2n+1)& =d(n),\end{array}$$ and define Toep­litz matrices $A,B,C,D$ (in­fin­ite on both sides) by $A_{nk}=a(n-k),\dots$, and func­tions $\alpha ,\beta ,\gamma ,\delta$ by $\alpha (x)=\sum _{n}a(n)e^{inx}$, …, which are en­tire since only fi­nitely many $a,b,\dots$ are nonzero, then the con­di­tions be­come $$\begin{array}{rlrl}\{\begin{array}{l}{A}^{\ast }A+C^{\ast }C=\text{𝟙}\\ B^{\ast }B+D^{\ast }D=\text{𝟙}\\ A^{\ast }B+C^{\ast }D=0\end{array}& \text{or}\{\begin{array}{l}|\alpha (x){|}^2+|\gamma (x){|}^2=1\\ |\beta (x){|}^2+|\delta (x){|}^2=1\\ \overline{\alpha (x)}\beta (x)+\overline{\gamma (x)}\delta (x)=0\end{array}& & (1)\leftrightarrow (1^{\prime })\\ \\ A^{\ast }C+B^{\ast }D=0& \text{or}\overline{\alpha (x)}\gamma (x)+\overline{\beta (x)}\delta (x)=0.& & (2)\leftrightarrow (2^{\prime })\end{array}$$ It fol­lows from these last two equa­tions (this is most eas­ily seen on the $\alpha ,\beta ,\gamma ,\delta$ side) that $$|\beta (x){|}^2=|\gamma (x){|}^2,\text{or}{B}^{\ast }B=C^{\ast }C,$$ and hence $$|\alpha (x){|}^2=|\delta (x){|}^2,\text{or}{A}^{\ast }A=D^{\ast }D.$$ Let $n_a,n_b,n_c,n_d$ be the widths of the di­ag­on­al bands out­side which the Toep­litz matrices $A,B,C,D$ van­ish. Then, since $B^{\ast }B=C^{\ast }C$ and $A^{\ast }A=D^{\ast }D$, we have $$n_b=n_c,n_a=n_d,$$ and, if $n_a,n_b,n_c,n_d$ are all nonzero, $$n_a=n_c,n_b=n_d.$$ (This be­cause the width of $B^{\ast }B$ is $2n_b-1$, that of $D^{\ast }D$ is $2n_d-1$, and $B^{\ast }B+D^{\ast }D=\text{𝟙}$.) We there­fore have $$n_a=n_b=n_c=n_d.$$

Sup­pose there ex­ist wave­lets as­so­ci­ated to $f,g$. Sup­pose that $\phi$ is sym­met­ric about 0, as in all in­ter­est­ing cases up to now. Then $f(n)$ is sym­met­ric about 0: $f(-n)=f(n)$. If there are only fi­nitely many nonzero $f(n)$, this im­plies that $$n_a\ne n_b,$$ ($n_a+n_b$ is ne­ces­sar­ily odd in this case).

It fol­lows that there are no com­pactly sup­por­ted or­thonor­mal wave­let bases with $\phi$ sym­met­ric about 0 and com­pactly sup­por­ted.

In the case of the Haar basis, $\psi$ is sym­met­ric about $\frac{1}{2}$. Since sym­metry about 0 is not pos­sible, what about sym­metry about $\frac{1}{2}$? This leads to $$f(n+1)=f(-n)$$ or $$C=A^{\ast }\text{(let’s suppose the }f\text{ are real)}.$$ But then $2A^{\ast }A=\text{𝟙}$, which im­plies $$n_a=1.$$ The solu­tion, unique up to per­muta­tions and shifts, is then $$A=C=\frac{1}{\sqrt{2}}\text{𝟙},B=-D=\frac{1}{\sqrt{2}}\text{𝟙},$$ which cor­res­ponds to the Haar basis. There is no oth­er com­pactly sup­por­ted or­thonor­mal wave­let basis as­so­ci­ated to a com­pactly sup­por­ted func­tion $\phi$ sym­met­ric about $\frac{1}{2}$, apart from the Haar basis.

On the oth­er hand, if we drop the sym­metry re­quire­ment, there are non­trivi­al ex­amples.

For $n_a=n_b=n_c=n_d=1$, for ex­ample, we find that, for every $\lambda ,\mu \in \mathbb{R}$, the matrices $$A=N^{-1}(\lambda \mu +U),B=N^{-1}(\lambda -\mu U),C=N^{-1}(\mu -\lambda U),D=N^{-1}(1+\lambda \mu U),$$ where $N=[(1+{\lambda }^2)(1+{\mu }^2){]}^{1/2}$ and where $U$ is the “shift” mat­rix, $U_{nk}={\delta }_{n-k+1}$, sat­is­fy equa­tions $(1^{\prime })$ and $(2^{\prime })$.

If we also im­pose (3), then $\mu =\frac{\lambda -1}{\lambda +1}$, and $$\hat{\phi }(\xi )=\prod _{j=1}^{\mathrm{\infty }}[F(2^{-j}\xi )]$$ con­verges, and lies in $L^2(\mathbb{R}),$ where $$F(\xi )=\frac{1}{2(1+\lambda {)}^2}[\lambda (\lambda -1)+\lambda (\lambda +1)e^{i\xi }+(\lambda +1)e^{2i\xi }-(\lambda -1)e^{3i\xi }].$$ The func­tion $\phi$ is sup­por­ted in the in­ter­val $[0,3]$ (or in $[-1,2]$ if we ap­ply a “shift”). Al­to­geth­er it looks kind of funny. For $\lambda =2\pm \sqrt{3}$, $\hat{\phi }$ de­creases faster than $(1+|\xi |{)}^{-(1+\epsilon )}$, and there­fore $\phi$ is con­tinu­ous.

If we draw a graph, it looks like this:

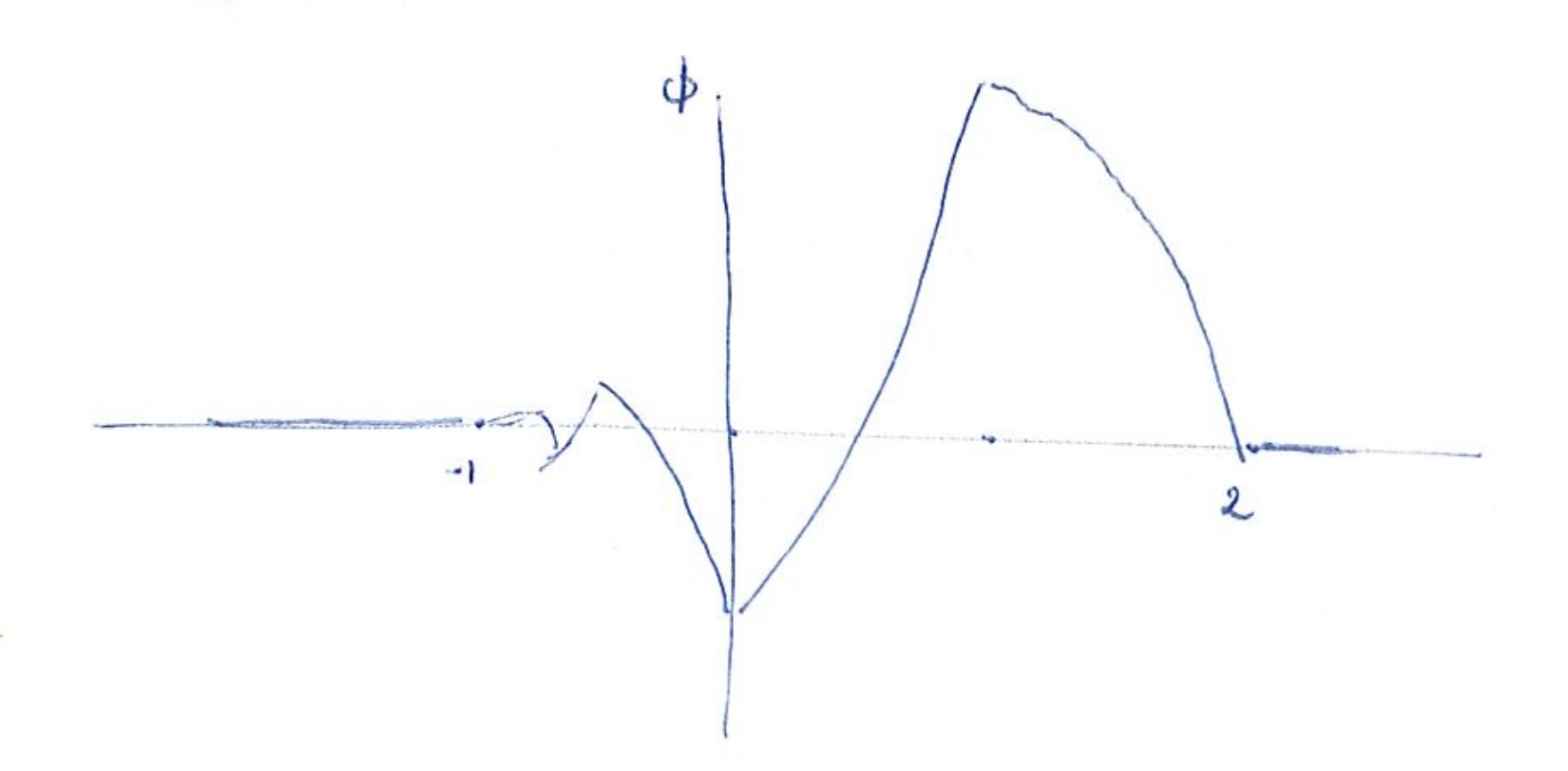
If we move on to $n_a=2$, we can find ex­amples of “cusp­less” $\phi$. My con­jec­ture now is that there ex­ist $\phi \in C^k$ in the class $n_a=k+1$.

I had ex­pec­ted that the “vis­ion people” would love a pyr­am­id al­gorithm re­cur­rence with a fi­nite num­ber of terms, but they’re not very fond of the asym­metry. But per­haps the con­struc­tion might serve for oth­er things…?

That’s all the news I have.

My best greet­ings to Anne, and to Guy Dav­id!

In­grid

$$\star \star \star$$

Cher Yves,

Com­ment vas-tu? Il y a longtemps que je voulais t’écri­re, et j’ai un peu honte d’avoir at­tendu si longtemps. Mais voilà en­fin de mes nou­velles.

Ann Ar­bor nous a fait, à Robert et moi deux of­fres de “as­so­ci­ate pro­fess­or­ships” (“with ten­ure”). Ils m’ont dit qu’ils étaiet fort im­pres­sionnés par ta lettre de re­com­mend­a­tion, pour laquelle je voudrais en­core te re­mer­ci­er. D’autre part, Bell Labor­at­or­ies aus­si m’a fait une of­fre (Robert y trav­aille déjà). Il nous faut décider av­ant le 1er mars (en­core une se­maine…). Si j’étais la seule à décdi­er, je crois que je pench­erais plutôt vers Ann Ar­bor: j’aime beau­c­oup l’en­droit, et c’est une bonne uni­versité. J’y ap­pren­drais beau­c­oup plus de math, aus­si. D’autre part, Robert est vraiment plus at­taché à Bell Labs que je ne croy­ais (et qu’il ne croy­ait lui-même d’ail­leurs), et, comme je répugne de com­men­cer notre mariage en le séparant de Bell al­ors qu’il s’y plaît, nous op­ter­ons prob­able­ment pour la solu­tion Bell. Je crois d’ail­leurs que je me plair­ai beau­c­oup à Bell aus­si: il y a de très bons mathématiciens là aus­si, et ils lais­sent une grande liberté à leurs cher­ch­eurs. Tu avais dit que, si nous al­lions à Ann Ar­bor, tu viendrais nous rendre vis­ite, Robert et moi et les petits. J’espère que tu viendras aus­si nous voir si nous pren­ons la voie Bell! Je crois que tu trouverais cer­tains des mathématiciens intéress­ants (An­drew Odlyzko, Ron Gra­ham, Larry Shepp, Jeff Lagari­as, Neil Sloane, …). Eux aus­si étaient fort im­pres­sionnés par ta re­com­mend­a­tion… Merci de m’avoir ouvert ces por­tes!

En at­tend­ant de décider tout à fait défi­nit­ive­ment (ce qui sera fait cette se­maine), j’ai en­fin ter­miné le gros papi­er, frames (dont j’avais vraiment marre à la fin), et je me suis re­mis aux ondelettes. L’ar­rivée du papi­er de Stéphane Mal­lat, que je trouve très beau, m’a amenée a réfléchir sur les bases or­thonor­males d’ondelettes. Je me de­mandais aus­si si un al­gorithme en “pyr­am­ide” comme dans le pa­per de Mal­lat, néces­saire­ment devait re­poser sur une base d’ondelettes. J’en ai dis­cuté avec des “vis­ion”-peuple ici, et ils semblent penser qu’une pro­priété d’or­tho­gon­alité des sous-es­paces de ${\ell }^2(\mathbb{Z})$, qui al­ors im­pose l’ex­ist­ence d’une base or­thonor­male d’ondelettes sous-ja­cent la “pyr­am­ide”, est es­sen­ti­elle. Tout cela m’a aus­si amenée à la con­struc­tion de bases or­thonor­males d’ondelettes à sup­port com­pact. (Voilà que moi, qui étais tou­jours dans les frames, je sombre dans l’or­thonor­mal aus­si!) Elles ex­ist­ent, mais les ex­emples que j’ai trouvés ont une drôle de gueule.

L’idée est la suivante.

Es­say­ons de con­stru­ire des suites $f(n),g(n)$ tell­es que $$\begin{array}{}\text{(1)}& \sum _{k}f(n-2k)f^{\ast }(\ell -2k)+g(n-2k)g^{\ast }(\ell -2k)={\delta }_{n\ell }.\end{array}$$ (au fond, c’est tout ce qui est néces­saire pour qu’une “pyr­am­ide” de décom­pos­i­tion $+$ re­con­struc­tion de ${\ell }^2(\mathbb{Z})$ marche.)

On peut al­ors définir $G,F:{\ell }^2(\mathbb{Z})\to {\ell }^2(\mathbb{Z})$ par $$(Fc{)}_k=\sum _{n}f(n-2k)c_n,(Gc{)}_k=\sum _{n}g(n-2k)c_n,$$ Al­ors $F^{\ast }F+G^{\ast }G=\text{𝟙}$, et on a $$(F^{\ast }{)}^N{F}^N+\sum _{j=1}^N{F}^{\ast N-j}{G}^{\ast }G{F}^{N-j}=\text{𝟙},$$ c.à.d. une décom­pos­i­tion $+$ re­con­struc­tion avec al­gorithem en pyr­am­ide de ${\ell }^2(\mathbb{Z})$. En pratique, on choisira $f,g$ réels.

Il parait rais­on­nable (mais non pas néces­saire) de se re­streindre au cas où $$F^{\ast }{\ell }^2(\mathbb{Z})\perp G^{\ast }{\ell }^2(\mathbb{Z})$$ (il est clair que la somme des 2 es­paces est ${\ell }^2$ dans tous les cas). Cela mènerait (d’après l’in­tu­itions des vis­ion people) à une plus grande “data com­pac­tion que le cas non-or­tho­gon­al. On im­pose donc \ad­dto­counter{equa­tion}{1} $$\begin{array}{}\text{(1)}& \sum _{n}f(n-2k)g^{\ast }(n-2l)=0.\end{array}$$

Toutes ces con­di­tions sont évidem­ment sat­is­faites si les $f,g$ sont ob­tenus à partir d’une base d’ondelettes. D’autre part, si $$\begin{array}{}\text{(2)}& \sum _{n}f(n)=\sqrt{2},\sum _{n}g(n)=0\end{array}$$ (ces 2 con­di­tions sont équi­val­entes si on a toutes les autres), et si $$F(\xi )=\frac{1}{\sqrt{2}}\sum _{n}f(n)e^{in\xi }$$ sat­is­fait que $$\prod _{j=1}^{\mathrm{\infty }}F(2^{-j}\xi )\in L^2(\mathbb{R}),$$ al­ors on a une base d’ondelettes as­sociées: $$\begin{array}{c}\hat{\psi }(\xi )=\prod _{j=1}^{\mathrm{\infty }}F(2^{-j}\xi ),\psi (x)-\sum _{k}g(k)\sqrt{2}\phi (2x-k),\\ ⟨{\phi }_{-1k},{\phi }_{0n}⟩=f(n-2k),\\ ⟨{\psi }_{-1k},{\phi }_{0n}⟩=g(n-2k).\end{array}$$ Si on im­pose, au départ, que les suites $f(n),g(n)$ n’ont qu’un nombre fini de terms non-nuls, al­ors les $\psi ,\phi$ as­sociés auront un sup­port com­pact ($F$, et par conséquent $\prod _{j=1}^{\mathrm{\infty }},F(2^{-j}\xi )$, est, dans ce cas-là, en­ti­er de type ex­po­nen­tiel).

ll s’agit donc de trouver des $f,g$ sat­is­fais­ant toutes les con­di­tions.

Si on défi­nit $$\begin{array}{rlrl}f(2n)& =a(n),& g(2n)& =c(n),\\ f(2n+1)& =b(n),& g(2n+1)& =d(n),\end{array}$$ et des matrices Toep­litz $A,B,C,D$ (in­finies des 2 côtés) par $A_{nk}=a(n-k),\dots$, et des fonc­tions entières (puisque un nombre fini seule­ment des $a,b,\dots$ est $\ne 0$) $\alpha ,\beta ,\gamma ,\delta$ par $\alpha (x)=\sum a(n)e^{inx}$, …, al­ors les con­di­tions devi­ennent $$\begin{array}{rlrl}\{\begin{array}{l}{A}^{\ast }A+C^{\ast }C=\text{𝟙}\\ B^{\ast }B+D^{\ast }D=\text{𝟙}\\ A^{\ast }B+C^{\ast }D=0\end{array}& \text{ou}\{\begin{array}{l}|\alpha (x){|}^2+|\gamma (x){|}^2=1\\ |\beta (x){|}^2+|\delta (x){|}^2=1\\ \overline{\alpha (x)}\beta (x)+\overline{\gamma (x)}\delta (x)=0\end{array}& & (1)\leftrightarrow (1^{\prime })\\ \\ A^{\ast }C+B^{\ast }D=0& \text{or}\overline{\alpha (x)}\gamma (x)+\overline{\beta (x)}\delta (x)=0.& & (2)\leftrightarrow (2^{\prime })\end{array}$$ Une conséquence de ces 2 dernières éq. est (cela se voit le mieux du côté $\alpha ,\beta ,\gamma ,\delta$ $$|\beta (x){|}^2=|\gamma (x){|}^2,\text{ou}{B}^{\ast }B=C^{\ast }C,$$ et donc $$|\alpha (x){|}^2=|\delta (x){|}^2,\text{ou}{A}^{\ast }A=D^{\ast }D.$$ Soi­ent $n_a,n_b,n_c,n_d$ la largeur de la bande di­ag­onale en de­hors de laquelle les matrices Toep­litz $A,B,C,D$ sont nulles. Al­ors, puisque $B^{\ast }B=C^{\ast }C$, $A^{\ast }A=D^{\ast }D$, $$n_b=n_c,n_a=n_d,$$ et, si tous les $n_a,n_b,n_c,n_d\ne 0$, $$n_a=n_c,n_b=n_d.$$ (Ceci parce que la largeur de $B^{\ast }B=2n_b-1$, celle de $D^{\ast }D=2n_d-1$, et $B^{\ast }B+D^{\ast }D=\text{𝟙}$). On a donc $$n_a=n_b=n_c=n_d.$$ Sup­po­sons qu’il ex­iste des ondelettes as­sociées aux $f,g$. Sup­po­sons que $\phi$ soit symétrique au­tour de 0, (comme dans tous les cas intéress­ants jusqu’à présent). Al­ors $f(n)$ est symétrique au­tour de $0:f(-n)=f(n)$. Si il y a un nombre fini de $f(n)$ non­nuls, cela im­plique $$n_a\ne n_b,$$ ($n_a+n_b$ est néces­saire­ment im­pair dans ce cas-ci).

Il n’y a donc pas de bases or­thonor­males d’ondelettes à sup­port com­pact avec $\phi$ de sup­port com­pact et symétrique au­tour de 0.

Dans le cas de la base de Haar, $\psi$ est symétrique au­tour de $\frac{1}{2}$. La symétrie au­tour de 0 étant ex­clue, qu’en est-il par la symétrie au­tour de $\frac{1}{2}$? Ceci mène à $$f(n+1)=f(-n)$$ ou $$C=A^{\ast }\text{(supposons les }f\text{ réels)}.$$ Mais al­ors $2A^{\ast }A=\text{𝟙}$, ce qui im­plique $$n_a=1.$$ L’unique solu­tion (à des per­muta­tions et das “shifts” près) est al­ors $$A=C=\frac{1}{\sqrt{2}}\text{𝟙},B=-D=\frac{1}{\sqrt{2}}\text{𝟙},$$ ce qui cor­res­ond à la base de Haar. Il n’y a pas d’autre base or­thonor­male d’ondelettes à sup­port com­pact as­socié à une fonc­tion $\phi$ de sup­port com­pact, au­tour de $\frac{1}{2}$, que la base de Haar.

Si on laisse tomber l’idée de symétrie par contre, il y a des ex­emples non trivi­aux.

Pour $n_a=n_b=n_c=n_d=1$, par ex­emple, on trouve que, pour tout $\lambda ,\mu \in \mathbb{R}$, les matrices $$A=N^{-1}(\lambda \mu +U),B=N^{-1}(\lambda -\mu U),C=N^{-1}(\mu -\lambda U),D=N^{-1}(1+\lambda \mu U),$$ où $N=[(1+{\lambda }^2)(1+{\mu }^2){]}^{1/2}$, et où $U$ est la matrice “shift”, $U_{nk}={\delta }_{n-k+1}$, sat­is­font les équa­tions $(1^{\prime })$ et $(2^{\prime })$.

Si on im­pose en plus (3), al­ors $\mu =\frac{\lambda -1}{\lambda +1}$, et $$\hat{\phi }(\xi )=\prod _{j=1}^{\mathrm{\infty }}[F(2^{-j}\xi )]$$ con­verge, et est dans $L^2(\mathbb{R})$, où $$F(\xi )=\frac{1}{2(1+\lambda {)}^2}[\lambda (\lambda -1)+\lambda (\lambda +1)e^{i\xi }+(\lambda +1)e^{2i\xi }-(\lambda -1)e^{3i\xi }].$$ La fonc­tion $\phi$ a comme sup­port l’in­ter­vale $[0,3]$ (ou $[-1,2]$ si on fait un “shift”). En général elle a une drôle de gueule. Pour $\lambda =2\pm \sqrt{3}$, $\hat{\phi }$ de­croît plus vite que $(1+|\xi |{)}^{-(1+\epsilon )}$, et $\phi$ est donc con­tin­ue.

Si on fait un graph­ique, on a

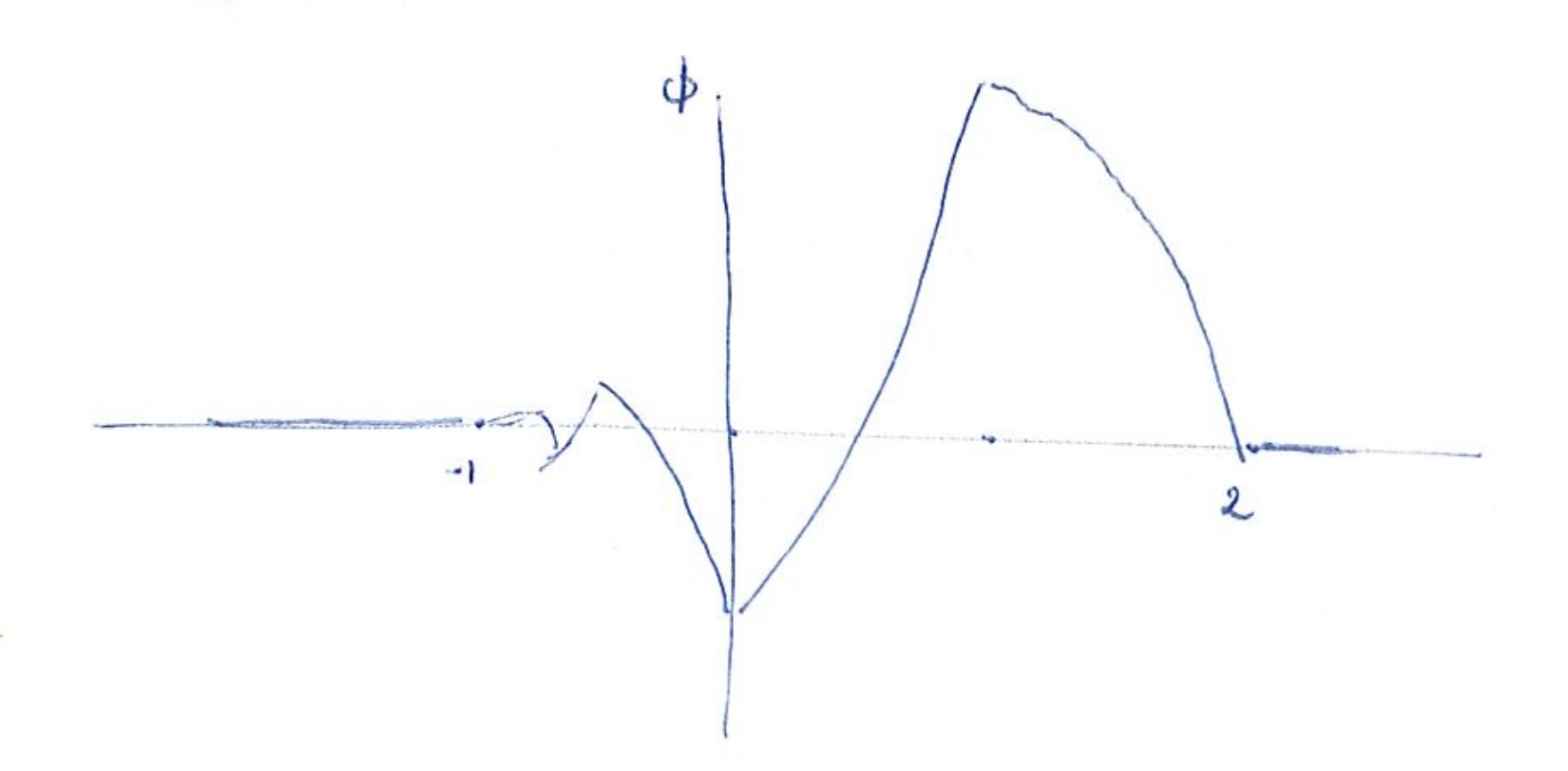
Si on passe à $n_a=2$, on ar­rive à trouver des $\phi$ sans “cusps”. Ma con­jec­ture, main­ten­ant, est qu’il ex­iste des $\phi \in C^k$ dans la classe $n_a=k+1$.

J’avais espéré que les “vis­ion people” aim­eraient une récur­rence pour l’al­gorithme pyr­am­id­al avec un nombre fini de ter­mes, mais ils n’aiment pas trop l’as­symétrie. Mais elles pour­raient peut-être ser­vir à autre chose…?

Voilà toutes mes nou­velles.

Un grand bon­jour à Anne, et à Guy Dav­id!

In­grid