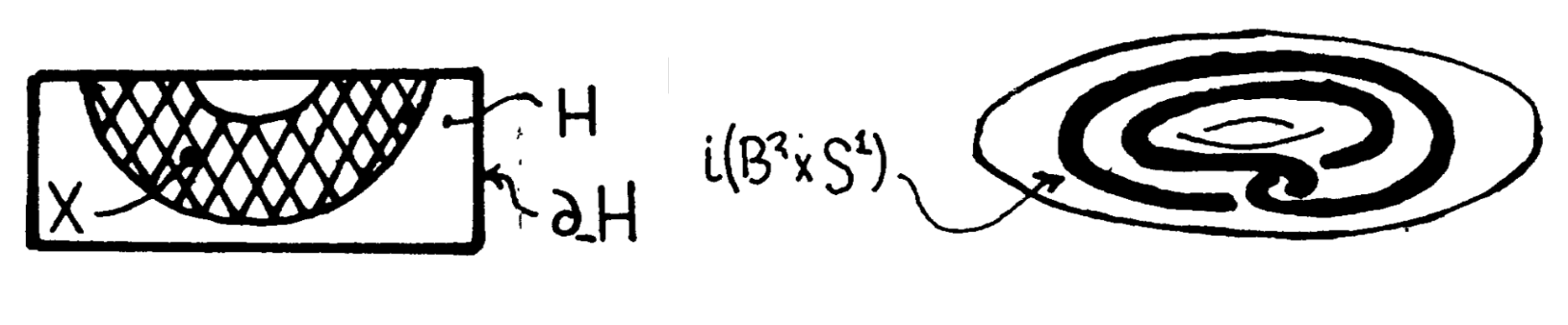by Laurent Siebenmann
French to English translation by Min Hoon Kim and Mark Powell
Introduction
At the end of the summer of 1981, in San Diego, M. Freedman proved that every
smooth homotopy 4-sphere
H. Poincaré
conjectured that every smooth, homotopy
J. Milnor
[e4]
discovered smooth manifolds
We sketch a proof of Freedman’s theorem which implies the topological
classification of smooth, simply connected
closed 4-manifolds and many
other results of
fundamental importance. Let
Proof.
It is not difficult to realise
In particular, we will prove the simply connected, topological 5-dimensional
For
Similarly, to obtain the fact that
Whitney
introduced a natural method for solving these problems. In the
model
We call a smooth (resp. topological) Whitney process, a smooth
embedding (resp. a topological embedding) of a disjoint union of copies of
the model
The first step of the proof (1973–1976) is due to
A. Casson.
Let
The theorem of Casson and Freedman now follows from the theorem that we will discuss.
The noncompact version of Theorem B is also important.
The difficult proof proposed by Freedman (October 1981) initiates the
proof of the proper
This gives (compare
[1]
and
[e34])
the topological
classification of closed, simply connected topological 4-manifolds that
admit (do they all?) a smooth structure in the complement of a point. They
are classified by their intersection form on
It also follows (see
[1],
[e34])
that every smooth homology
3-sphere
Report
Mike Freedman announced his proof of the topological Poincaré conjecture in August 1981 at the AMS conference at UCSB where D. Sullivan was giving a lecture series on Thurston’s hyperbolization theorem. His argument was very brilliant, but not yet completely watertight.
A large group of experts then formulated certain objections, which led to the statement of the approximation theorem (Theorem 5.1). However, Freedman already had in his head his trick of replication, and in a few days, his imposing formal proof was born.
In the meantime, R. D. Edwards had found a mistake in the shrinking arguments (see Section 4) and, being an expert in this method, had repaired the mistake even before pointing it out. (I think that he introduced in particular the relative shrinking arguments.) At the end of October 1981, Freedman explained the details of his proof, with charm and patience, at a special conference at University of Texas at Austin (the school of R. L. Moore) before an audience of specialists, including, in the place of honour, Casson and RH Bing, creators of the two theories essential in the proof.
This paper relates the proof given in Texas, with improvements
in detail added in behind the scenes. Already in 1981,
R. Ancel
[2]
had clarified and improved the complexities
in bookkeeping of the approximation theorem (Theorem 5.1). In particular, he was
able to reduce a hypothesis of Freedman demanding that the preimages of
the singular point constitute a null decomposition, showing that
My exposition (January 1982) does not seem to have changed essentially
from my memories of Texas. Only my construction of corrective 2-discs (the
1. Terminology
A decomposition
The set of connected components of a space
The manifolds and submanifolds mentioned will be (unless otherwise
indicated) smooth. For manifolds, we adopt the usual convention
([e29], Essay I);
in particular,
2. Casson tower and Freedman’s mitosis
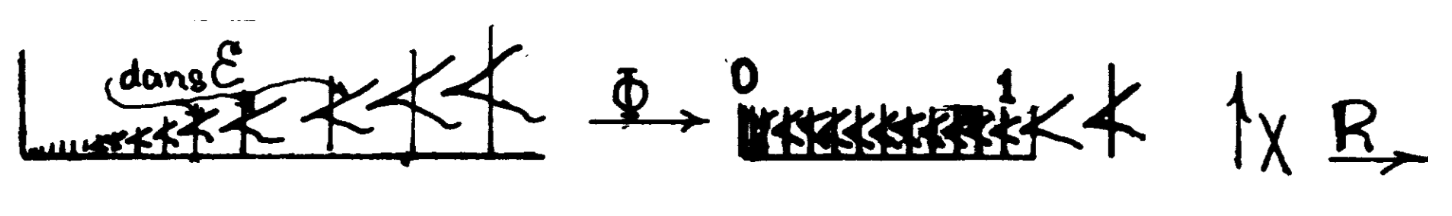
A defect
- in the 4-ball
A multidefect

Sketch of proof (see
[e38]).
If we attach a
multihandle
A residual defect
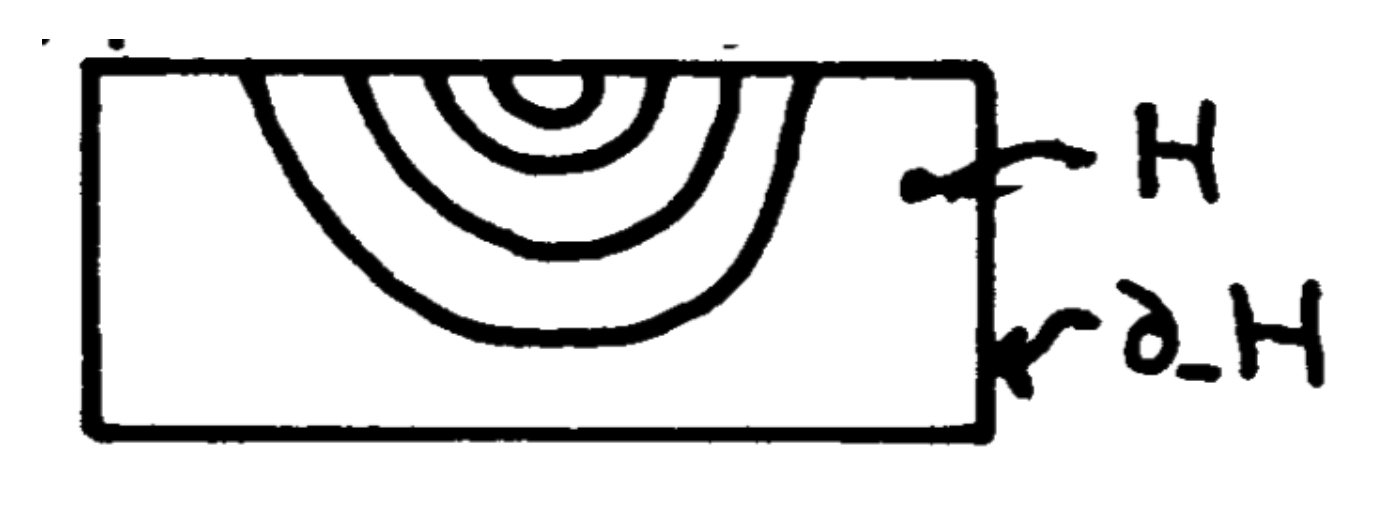
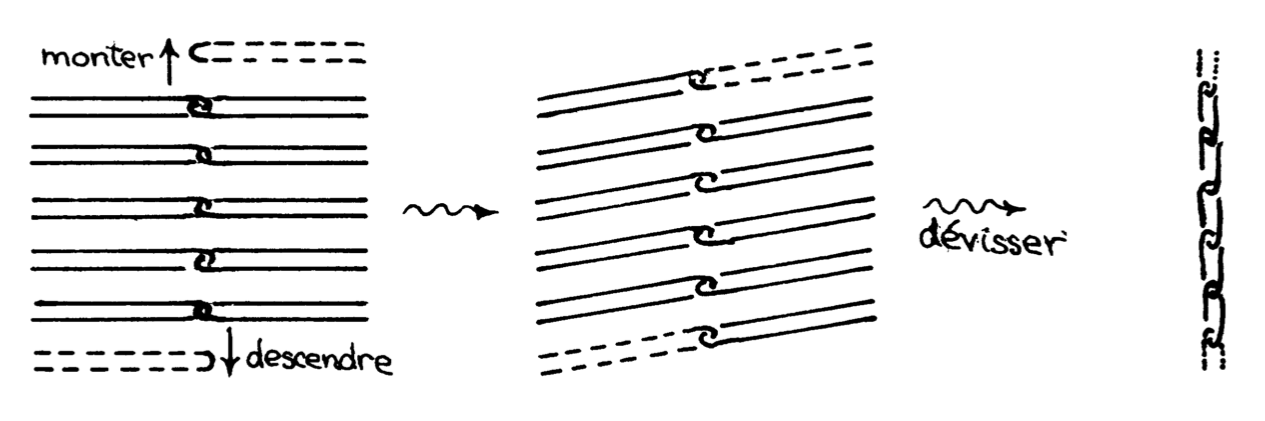
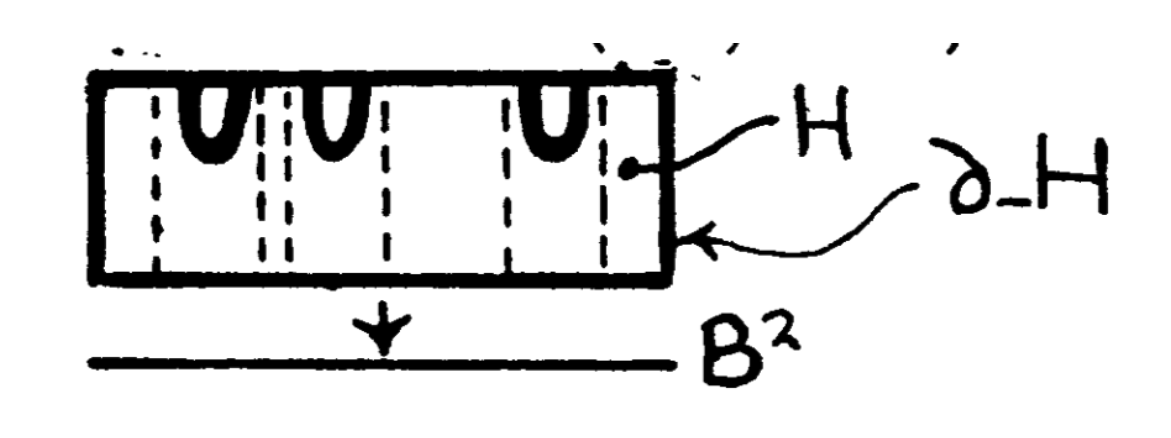
A Casson handle is a pair
The data of
The skin of of Similarly
A Casson handle
By
We remark that
The proof of Theorem 2.2 starts with a result of 1979, when Freedman was
able to construct a smooth 4-manifold
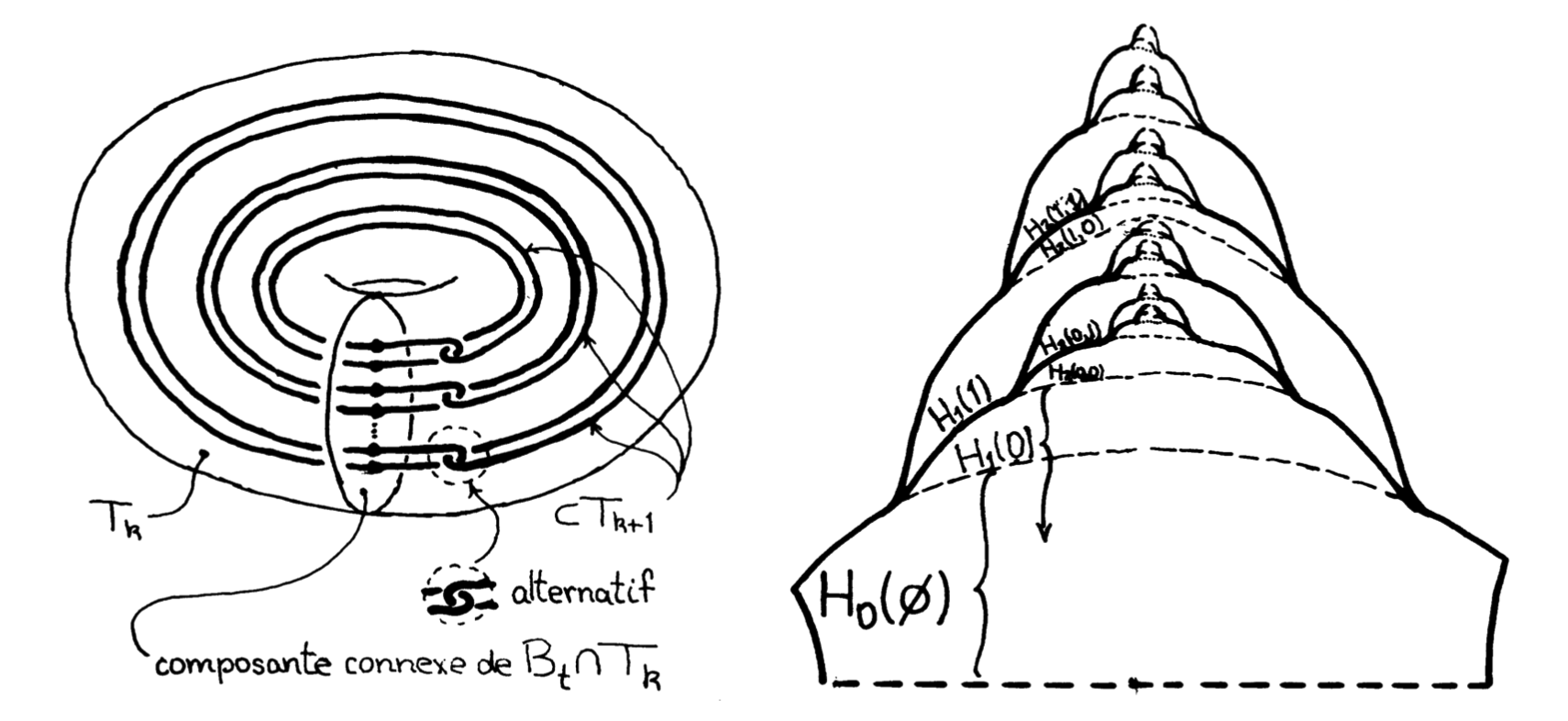
A Casson tower of height

Condition (3) is related to the fact that, for each Casson tower
In Section 3, Figure 6 will represent a

Figure 7.
The method of Freedman [1] (compare [e34]) allows one to give a proof of Theorem 2.3. However, it is slightly more detailed than the analogues in [1], [e34]. We will not cover this point in this paper (see [e37] for an excellent write up of the mitosis theorem (finite version, Theorem 2.3).
Since we are going to use Theorem 2.3 often, it is convenient to make the following:
- The closure
This infinite version, Theorem 2.5, follows from the finite version, Theorem 2.3, by an infinite repetition. One sufficiently shrinks balls given by Theorem 2.3 to ensure the condition (3) of Theorem 2.5.
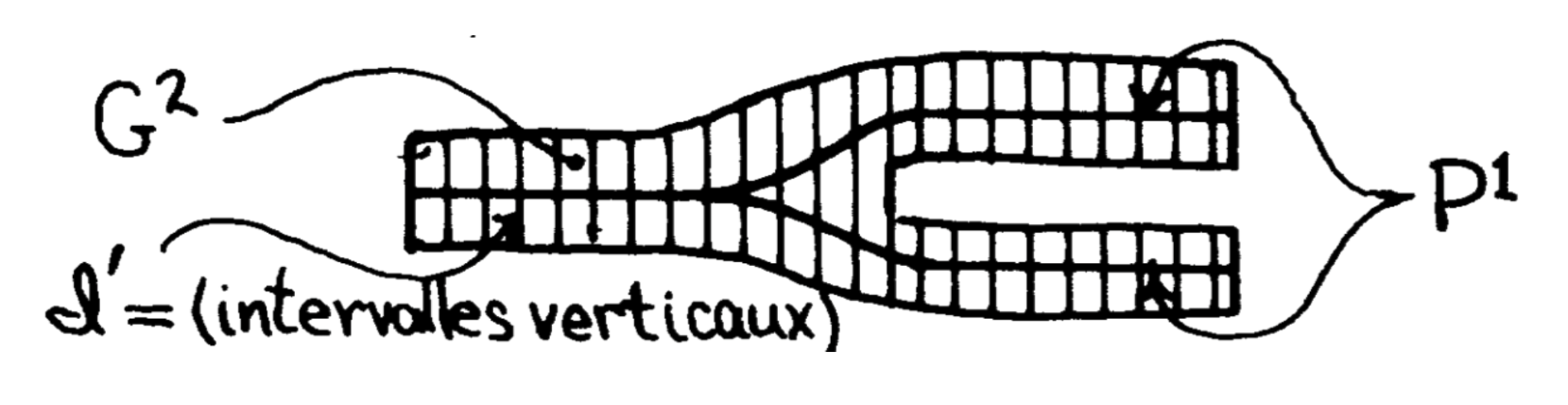
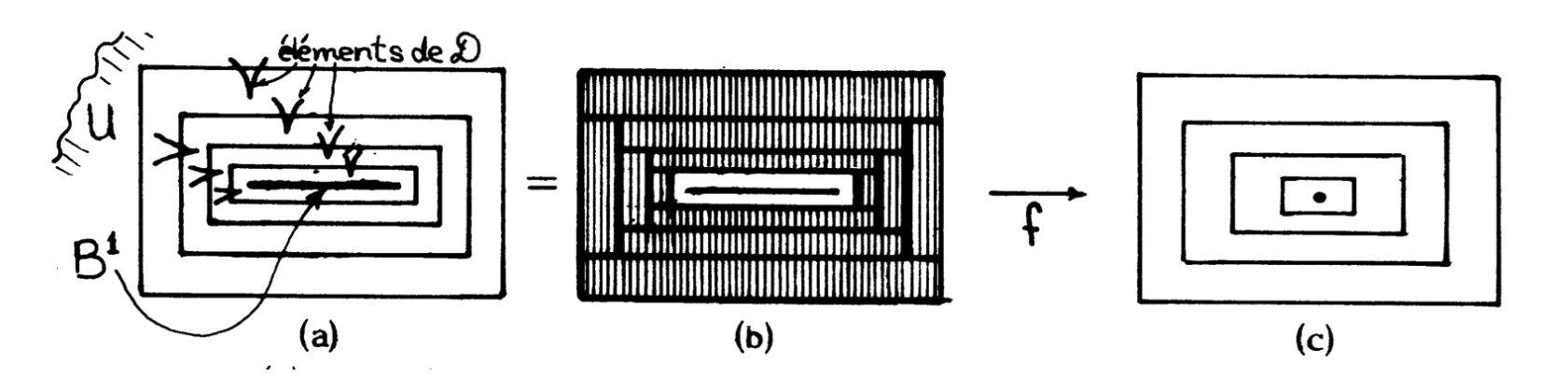
3. Architecture of topological coordinates
The open Casson handle
We will construct a ramified system of Casson handles in
3.1. Construction
- The closure
- The intersection of
- (Without Change of Notation 2.4)
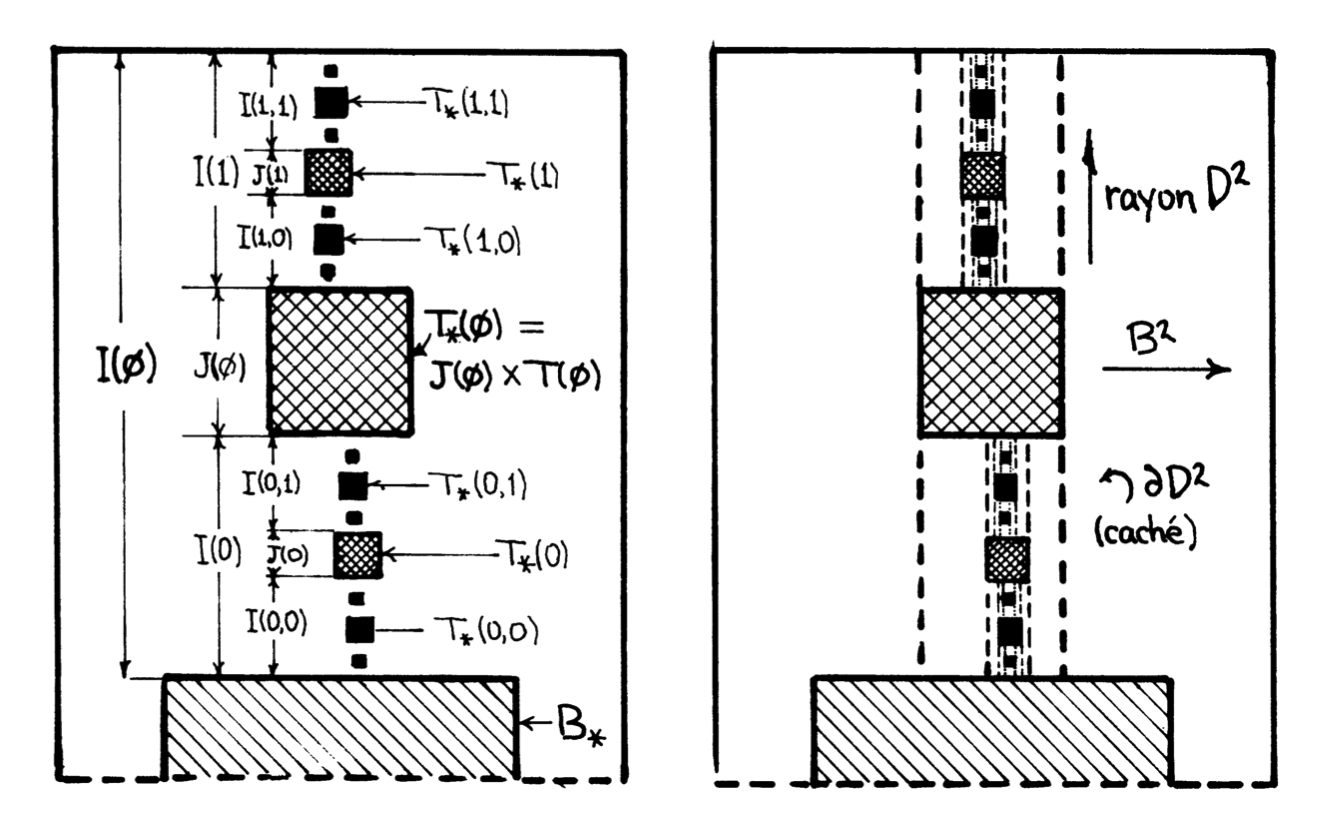
We also require a coherence condition on the total Russian doll assumed by
(7), that is to say
Execution of Construction 3.1 (by induction on
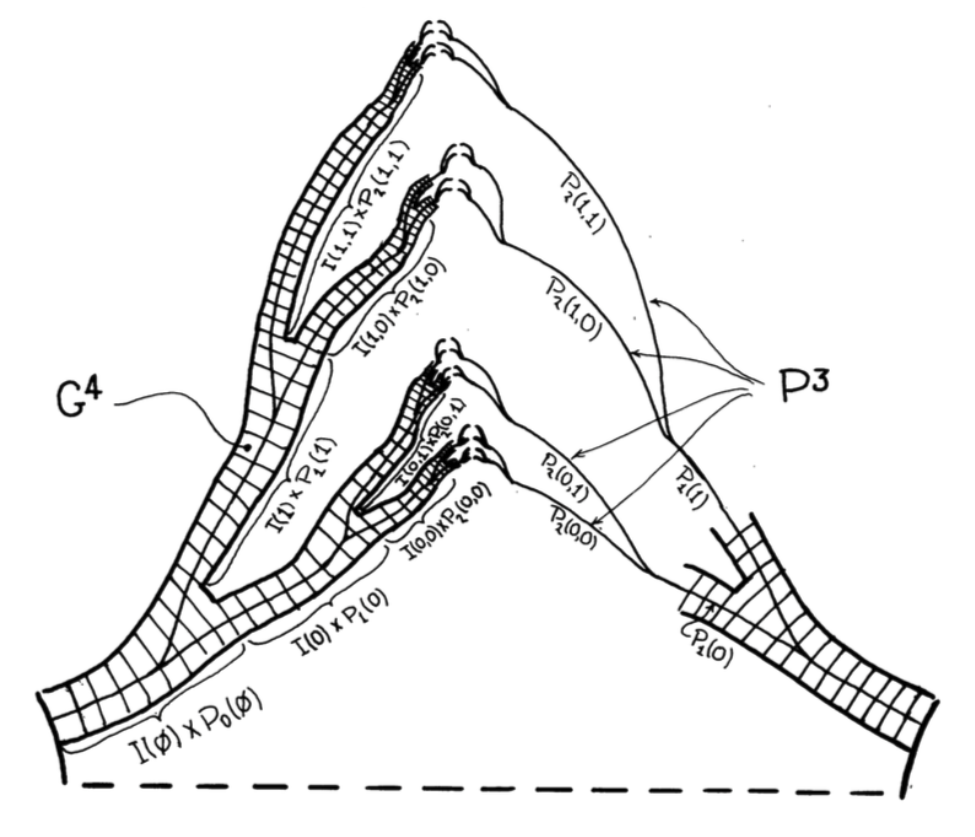
Of the system of handles
The branched manifold
3.2. Construction of the design
For
It follows by combining, quite naively, two bicollars of genuine submanifolds
of
The design
3.3. Construction of
This
The central third of
On the other hand, we claim that the
embeddings
We define
3.4. Construction of
3.5. The image
With this notation, we
claim that the image
3.6. The main diagram
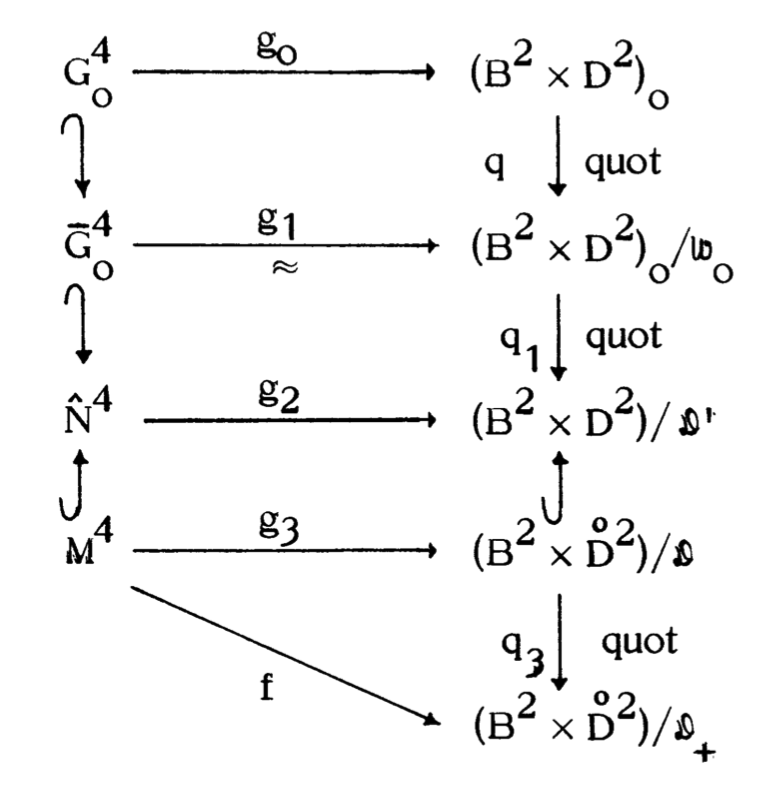
3.7. Construction of
3.8. Construction of
Next,
The decomposition
The construction of
3.9. Construction of
For the requirements of the next paragraph, the
quotient
- the boundary
- a multilongitude of
Moreover, we want that the diameter of the connected components of
The specification of
We have
To start, we specify (simultaneously and independently) in
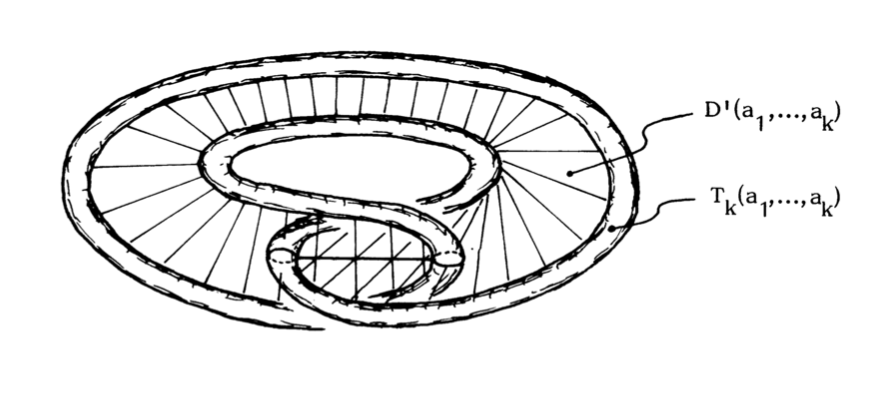
(a)
(b) For each
By resolving the double points of
We will embed a single
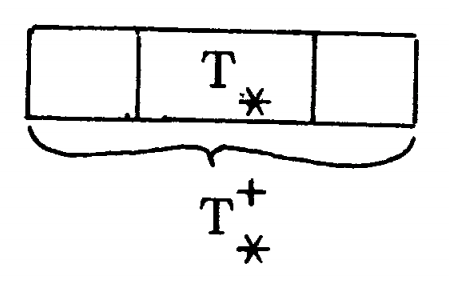
One can easily check that, for
(c)
(d) Each connected component of the multidisc
For
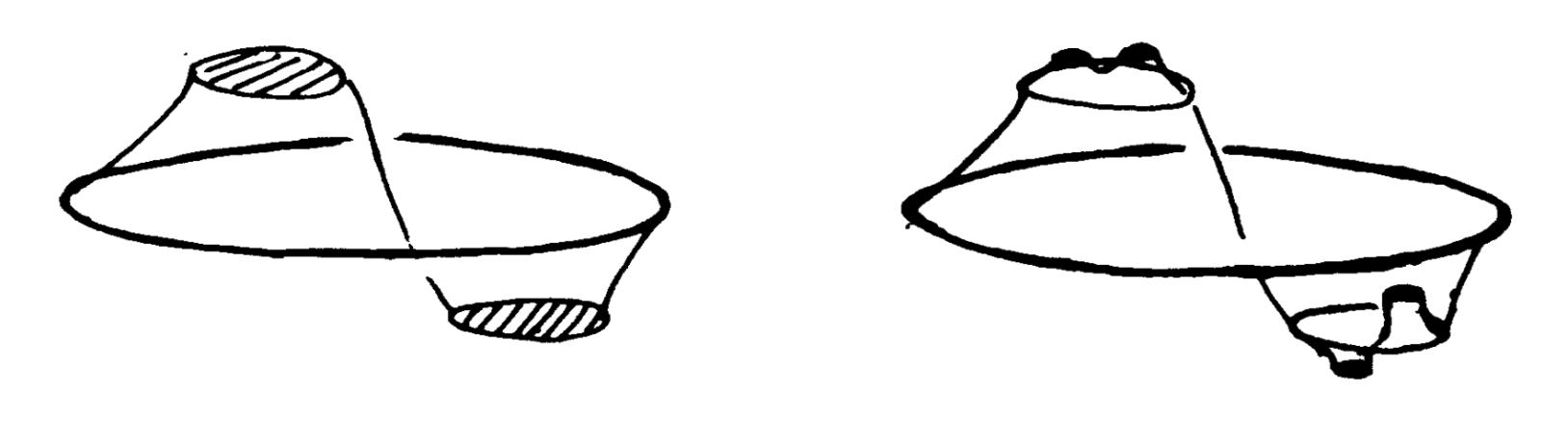
We observed that in pushing
(e) For each integer
This condition assures that, for all
Thus the family
3.10. End of the proof that
Also
Therefore, according to Theorem 5.1, the map
4. Bing shrinking
We consider a proper surjective map
Since
We then say that
Proof (indication).
To approximate
This principle is easy to establish, because if
This condition, modulo localisation principle (Proposition 4.2), is clearly necessary.
For all
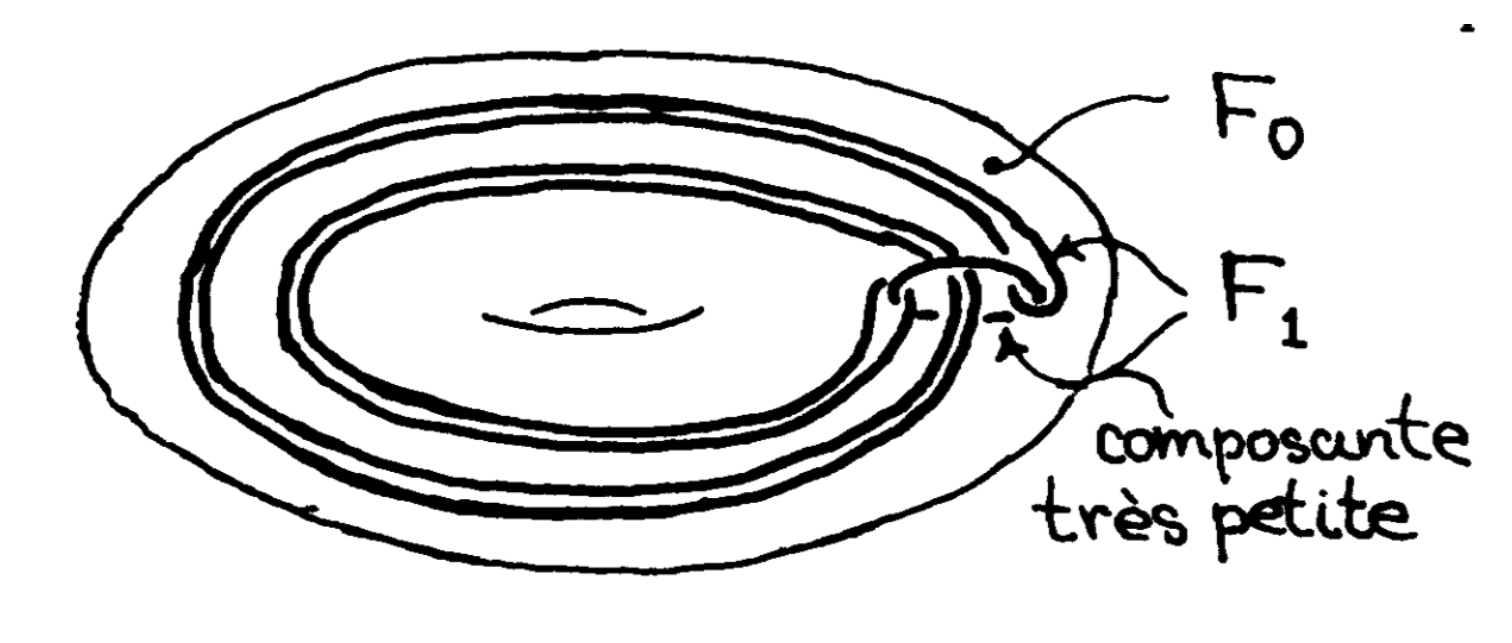
There are thankfully properties of individual elements, a little stronger than
cellularity, which discards this sort of example. For a compact
Proof. The proof is an edifying exercise. ◻
Proof of Proposition 4.6.
This is
In (a), every element of
By this lemma, one can shrink many decompositions related to Whitehead
compacta. For example, let
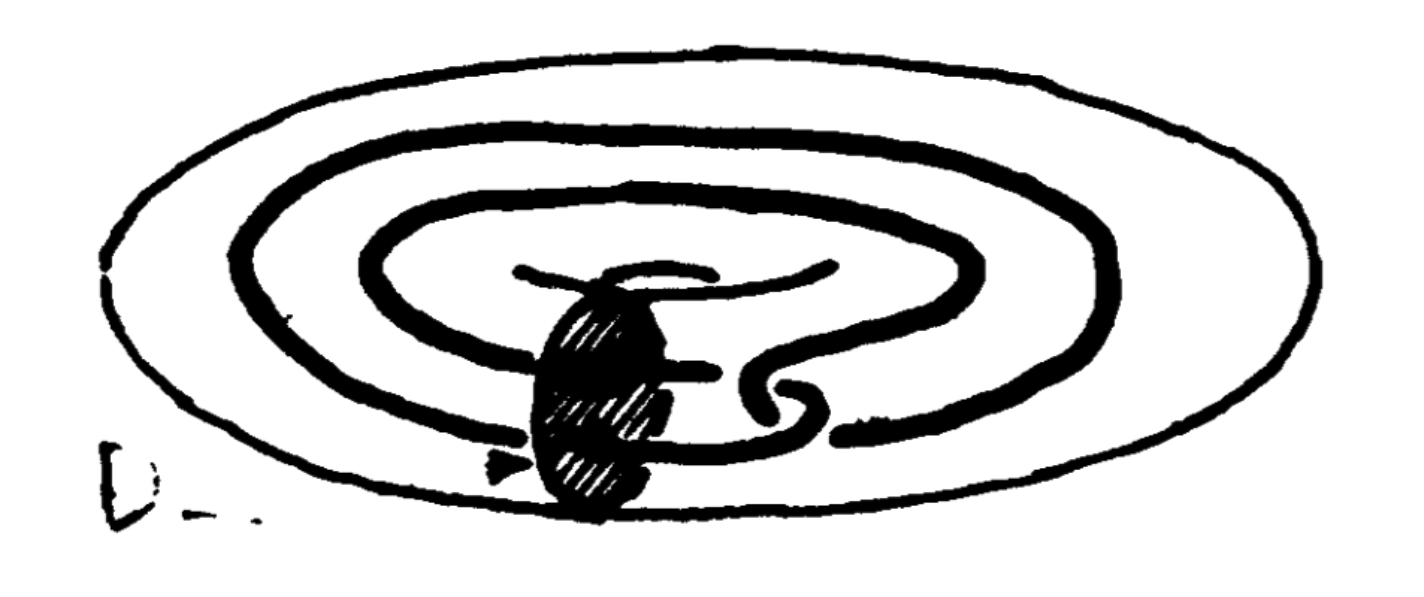
This is a result of
Andrews
and
Rubin
[e16]
in 1965, proved after
analogous results, but more difficult, of
Bing
[e5]
in 1959,
which is a curious anachronism. There is a good explanation!
A. Shapiro,
at the time when he succeeded in turning
To establish the flatness of the discs
Proof of Lemma 4.9.
This has nothing to do with the proof of
Lemma 4.7! We find
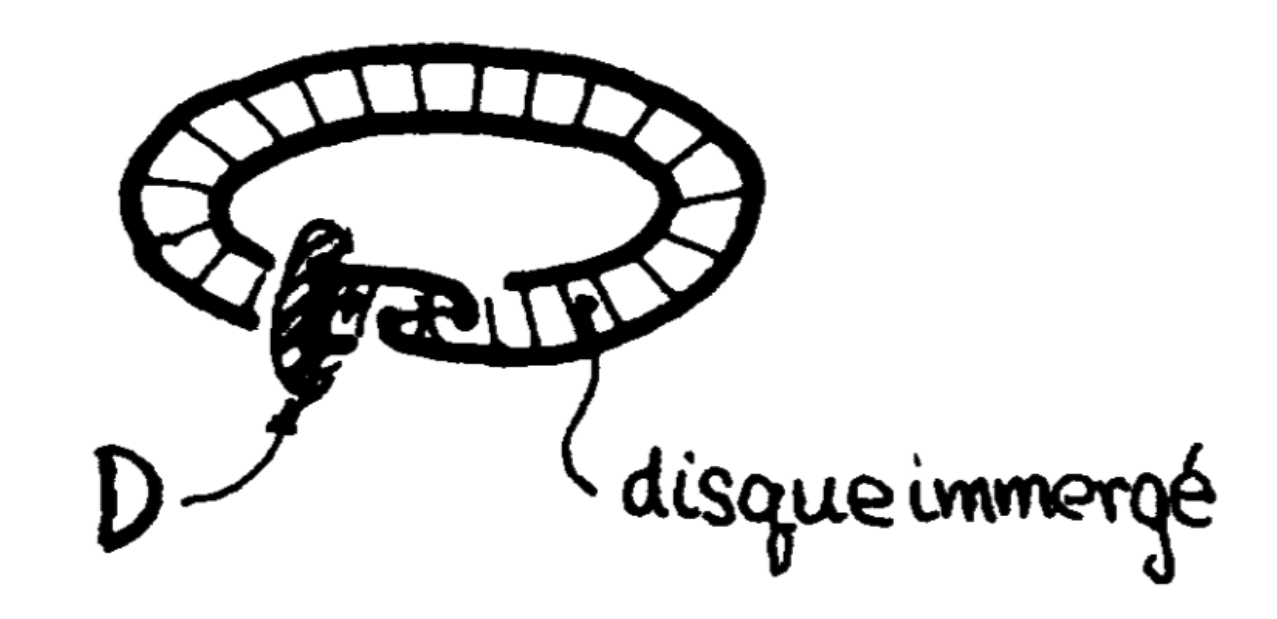
To establish that
Proof of Proposition 4.10.
We apply Theorem 4.4, Lemma 4.7 (or
Lemma 4.9, without exploiting the last condition of Lemma 4.9). For this,
it is convenient to remark first that for all open
Our next goal is the flatness of the
discs
Proof of Proposition 4.11.
We apply Lemma 4.9 and the relative
criteria (Theorem 4.4). For every open
Condition (d) of Section 3.9 allows us to choose these sets so that in addition, for each:
By condition (b) of Section 3.9 the meridional disc
- each intersection
For all compact
Proof of Proposition 4.12.
The open
set
We now propose to finish by showing that the quotient
maps
Proof of Proposition 4.13. This follows from Propositions 4.12, 4.6 and 4.5. ◻
To approximate
The decomposition
This will result in the following proposition.
Proof of Proposition 4.15.
This is equivalent to the existence
of a bicollar neighbourhood in
Proof of Proposition 4.14.
The map
Proof of Proposition 4.16.
Given an open neighbourhood
Proof of Lemma 4.17 (Indications.) Figure 21 completes the proof. □
All elements of
Proof of Proposition 4.18 (indications).
The quotient of
Proof. Apply Propositions 4.18, 4.16 and 4.5. ◻
5. Freedman’s approximation theorem
In the case that
Recall that a compact set
Compare the Bing shrinking criterion, Theorem 4.1
[e8].
A direct
proof shrinks
Proof of Theorem 5.1 if
In the setting of Freedman’s ideas, the case where
Proof of Theorem 5.3 (starting from Theorem 5.1 for
Shrinking
To prove Theorem 5.1, Freedman introduced a nice trick of iterated
replication of the approximation map, which vaguely reminds me of the
arguments of
Mazur
[e7].
This trick leads us to leave the
category of continuous maps and to instead work in the less familiar realm
of closed relations. It was during the seventies that closed relations
imposed themselves for the first time on geometric topology; they surfaced
implicitly in a very original article by
M. A. Stanko
[e24]
and have become essential since: I believe that it would be a herculean
task to prove, without closed relations, the subsequent theorem of
Ancel
and
Cannon
[e30]
that any topological embedding
A continuous map
By extending usual notions for continuous functions, for
- the image
- the restriction
- the inverse
To exploit an analogy between a function
Proof of Theorem 5.1.
Any submanifold of codimension 0 that
is introduced will be assumed to be topological and locally flat. Let
By removing a small
It is easy to see that Theorem 5.4 implies Theorem 5.1 using the special
case of Theorem 5.3 (Schönflies theorem) where
The first step of an inductive construction of
Suppose that
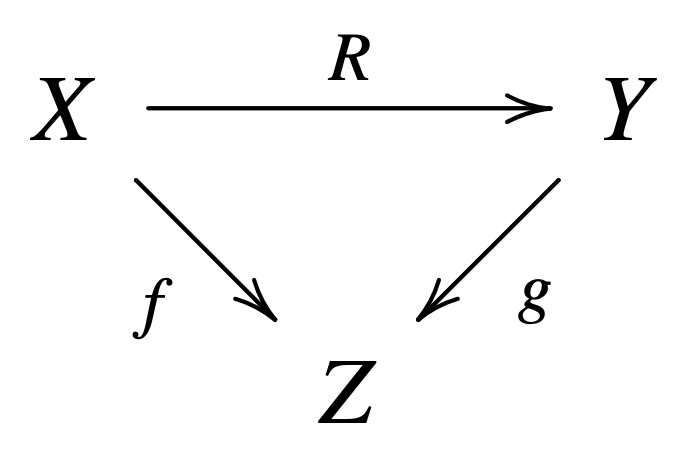
It is said that a good relation
- The singular sets
Then, for all
Proof of Addendum.
If the conclusion is false, then
there are two sequences of points of
Proposition 5.5 (with Addendum) will be used as a machine that swallows
the data
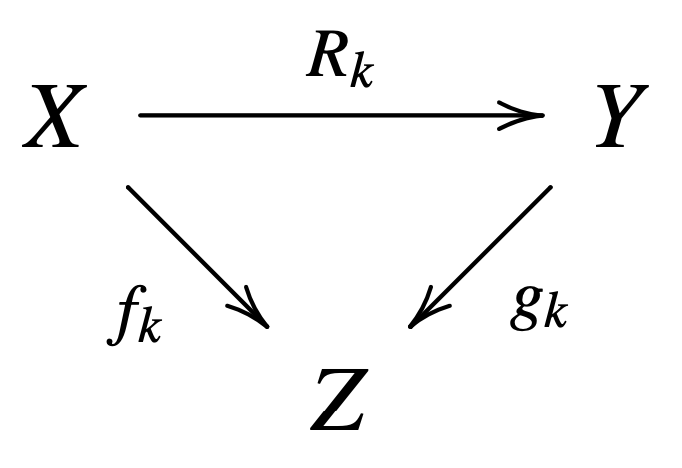
Let us continue constructing the homeomorphism
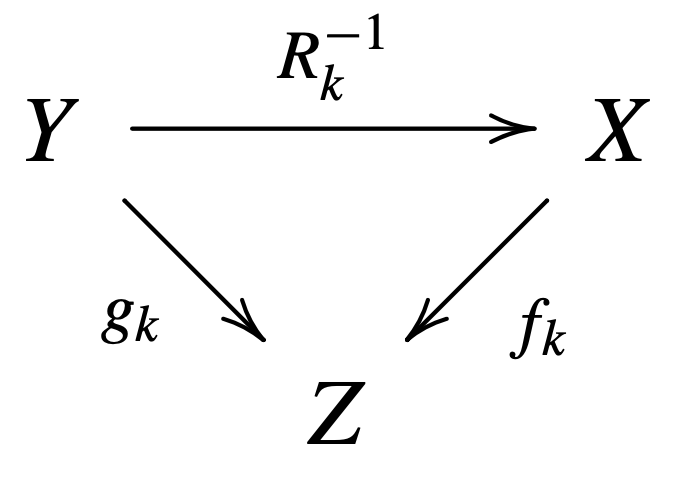
Suppose that the
- If
- If
By induction, we have
Proof of Proposition 5.5.
To explain the essential idea of
Freedman, the reader should read the proof with a view to (re)proving that a
surjection
Consider the preimages
Proof of Lemma 5.6
Consider the space
Then, the famous Baire category theorem asserts that the countable
intersection
- Each connected component
Proof of Claim 5.7.
Identify
In
(4)
We set
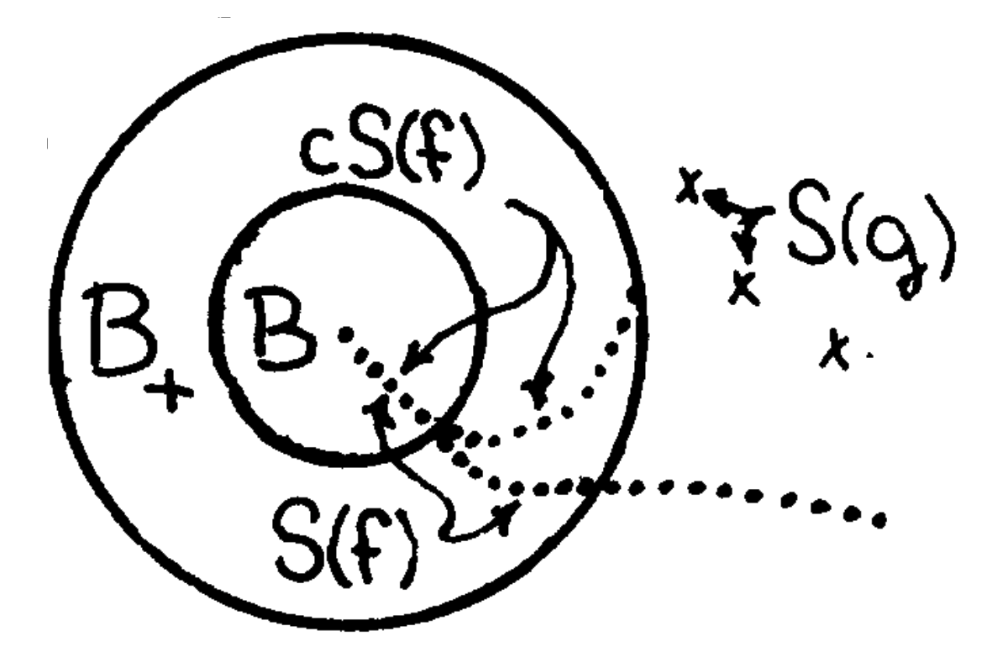
Let
Since
We have now
specified the modification
Verifying the claimed properties for