| [letterhead] | |
| The University of Michigan | |
| Department of Mathematics | |
| Ann Arbor, Michigan 48109-1003 | |
| Tel.: (313) 764-0335 | |
| Fax: (313) 763-0937 | |
| [handwriting] April 12, ’90 |
Dear Yves,
I’ve been promising myself to write to you for a while…and I’ve finally found the time to do it! — Many thanks for all the things you’ve sent me these last few months. I absolutely love your book, and have used portions of it in the last part of my course (in particular Section III.11, “A comparison of Fourier and wavelets”, and the characterization of \( L^P (\mathbb{R}) \) spaces, § VI.2). But I fear that engineering students in the US will find it too mathematical for their liking… — Thanks also for sending your paper on wavelet bases on \( [0,1] \). That, too, I’ve explained to my class, since it’s such a beautiful idea — I hope you won’t hold this against me! The result hasn’t been published yet, but I thought it a pity not to tell them about such a lovely construction.
Robert and I went to Berkeley a month ago, to give talks and be interviewed. Many thanks for writing such a glowing letter of recommendation! I haven’t seen it, of course, but Alberto Grünbaum, their chairman, told me it made quite an impression. You asked me in one of your notes whether I’d like a job at Berkeley… The answer is “yes”. I love the place and have the feeling I could learn a whole lot there. Our problem is that from Robert’s point of view, Berkeley is not very attractive: there is no one in combinatorics in the math department there and no one in coding theory in the electrical engineering department, and those are the two fields he works in. For him, Ann Arbor, which seems about to repeat its offer of 3 years ago, would be much better. On the other hand he hesitates (and I too, but less) to leave Bell, where there is a lot less red tape, but where I think the freedom we now have (of choosing our research topics) will not last.… Robert doesn’t fully share that fear, which means this has been a subject of daily discussion! Fortunately no arguments…We’ve promised Berkeley and Ann Arbor to decide this summer.
My teaching obligations are almost finished here: two more weeks of classes. I loved teaching this course, but it took up much more time than I expected. But it also motivated me to fully understand the finer points of Beylkin–Coifman–Rokhlin (and so also of theorem \( T(1) \)) and other proofs à la Calderón–Zygmund, which is a good thing (I should have started on it sooner!). On the other hand I’d hoped to learn some stuff in numerical analysis and PDEs during my stay here, and I’ve just barely made a start in that direction. I have May and June left to pick people’s brains here and to find a nice, juicy application for wavelets (which is what I most want: I’m getting tired of lecturing about this splendid tool that doesn’t have actual applications — although BCR [Beylkin–Coifman–Rokhlin] is an application, and a beautiful one, there should be others!).
Meanwhile I haven’t done much research, alas… and so I don’t have many results. A few little remarks:
• Two-dimensional setups, with \[ \eqalign{ \psi_{j,k} (x) = 2^{j/2} \psi (A^j x - k) \qquad k&\in \mathbb{Z}^2,\, j \in \mathbb{Z},\cr A&=\bigl(\textstyle{\phantom{-}0 \atop -1}{1 \atop 0}\bigr) , } \] can never have \( \psi \in C^1 \) (because \[ \frac{\partial}{\partial \xi_1} m_0 (\xi_1, \xi_2)|_{\xi_1 = \pi=\xi_2} = 0 = \frac{\partial}{\partial \xi_2} m_0 (\xi_1,\xi_2)|_{\xi_1=\pi=\xi_2} \] leads to \[ m_0 (\xi_1,\xi_2) = (1 + e^{i\xi_1})(1+e^{i\xi_2}) \mathcal{F} (\xi_1, \xi_2), \] which makes \[ |m_0 (\xi_1, \xi_2)|^2 + |m_0 (\xi_1 + \pi, \xi_2 + \pi)|^2 = 1 \] impossible).
• For noninteger but rational \( \alpha \), the right generalization of multiresolution analysis (I think) should use several \( \phi \) (and not just one). E.g., for \( \alpha = \frac{3}2 \), it is not possible to construct a compactly supported multiresolution analysis by taking only one \( \phi \) (in fact it can be shown that \( \hat{\phi} \) must have an interval not contained in its support), but they exist if one chooses two \( \phi \)s (each having integral 1), corresponding to the 2 different kinds of points in the pyramid:
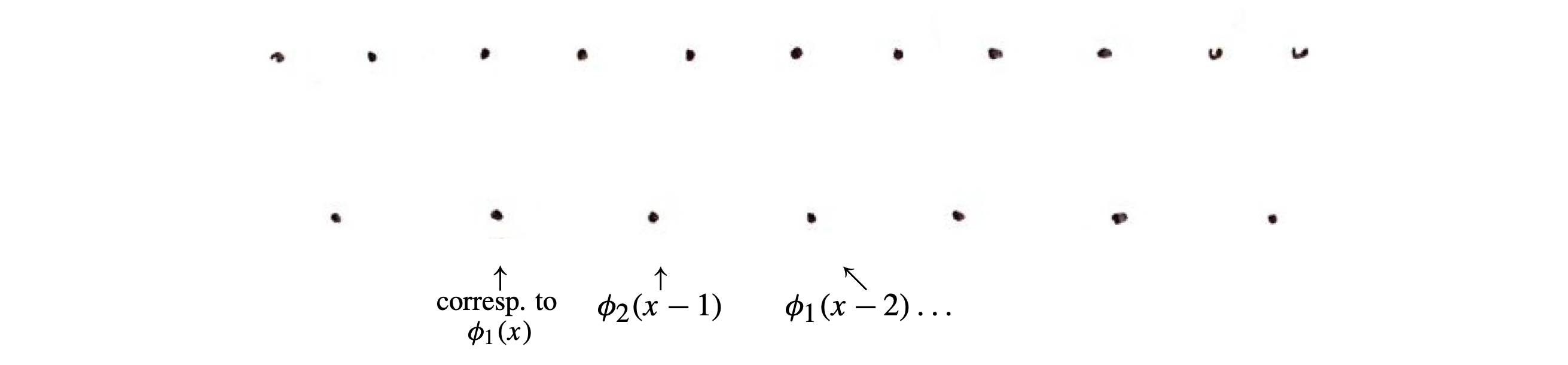
I have no idea how to impose regularity.
• I have a method for finding symmetric biorthogonal bases close to orthonormal bases. Its point of departure is the Battle–Lemarié wavelets, and it truncates \( m_0 \) to yield compact support. I’m now adding it to my article with A[lbert] Cohen and J. C. Feauveau (still not finished, to my great embarrassment! But it’s at the top of my agenda now that the teaching is under control), and I’ll send you and Albert details later.
That’s it for this letter!
My best to everyone,
Ingrid
\[ \star\qquad\star\qquad\star \]
Cher Yves,
Voilà longtemps que je me promets de t’écrire\ldots et je trouve
enfin le temps de le faire! — Merci beaucoup pour toutes les
choses que tu m’as envoyées ces derniers mois. J’aime énormément
ton livre, et j’en ai utilisé des sections dans la dernière partie
de mon cours (en particulier la section III.ii, “la confrontation
Fourier-ondelettes”, et la charactérisation des \( L^P
(\mathbb{R}) \), § VI.2). Je crois toutefois que les étudiants
ingénieurs aux E-U ne le trouvent trop mathématique à leur
goût\ldots — Merci aussi pour l’envoi de ton papier sur les
bases d’ondelettes sur \( [0,1] \). Là aussi j’ai expliqué l’idée,
que je trouve très belle, à ma classe — j’espère que tu ne
m’en veux pas! Le résultat n’est pas encore publié, mais je
trouvais dommage de ne pas leur parler de cette construction si jolie.
Robert
et moi sommes allés à Berkeley il y a un mois, pour donner
des exposés et être “interviewés”. Merci beaucoup d’avoir
écrit une si belle lettre de recommendation! Je ne l’ais pas vue,
évidemment, mais
Alberto Grünbaum,
leur chairman, m’a dit qu’elle
avait fait un grand effet. Tu me demandais dans une de tes notes si je
désirais avoir une position à Berkeley\ldots La réponse est
“oui”. J’aime beaucoup l’endroit et j’ai l’impression que je
pourrais y apprendre beaucoup. Le problème pour nous est que du
point de vue de Robert, Berkeley n’est pas très attirant: il n’y a
personne en “combinatorics” dans le département de math, et
personne en “coding theory” dans le département d’electrical
engineering, qui sont les deux domaines dans lesquels il travaille.
Pour lui, Ann Arbor, qui veut répéter son offre d’il y a 3 ans,
serait beaucoup mieux. D’autre part il hésite (moi un peu moins) à
quitter Bell où la vie bureaucratique est tellement plus facile,
mais où je crains que la liberté (de choisir notre sujet de
recherche) dont nous jouissons actuellement ne durera pas\ldots Robert
ne partage pas toutes mes craintes à ce sujet, ce qui fait que nous
discutons firme presque tous les jours! Heureusement sans nous
disputer\ldots Nous avons promis à Berkeley et à Ann Arbor de
décider cet été.
Ma charge d’ensignement est presque terminée ici: encore 2 semaines de cours. J’ai aimé donner ce cours, mais il m’a pris beaucoup plus de temps que je ne prévoyais. Mais il m’a aussi incité à vraiment comprendre tous les détails de Beylkin–Coifman–Rokhlin (et donc du théorème \( T(1) \)) et d’autres démonstrations Calderón–Zygmundesques, ce qui est une bonne chose (et à laquelle j’aurais du me mettre plus tôt!). D’autre part j’avais espéré apprendre des choses en analyse numérique et en PDE pendant mon séjour ici, et je n’ai fait que quelques petits pas dans ces directions-là. Il me reste mai et juin pour profiter des connaissances des gens ici, et pour trouver une belle application juteuse pour les ondelettes (ce qui est ce que je voudrais le plus: je commence à en avoir assez de donner un exposé sur cet outil magnifique sans une véritable application — bienque BCR [Beylkin–Coifman–Rokhlin] en soit une, et très belle, il en faudrait d’autres!).
Entre-temps je n’ai malheureusement pas fait beaucoup de
recherche\ldots Et je n’ai donc pas beaucoup de résultats. Quelques
petites remarques:
• Les schèmas en 2 dimensions, avec
\[
\eqalign{
\psi_{j,k} (x) = 2^{j/2} \psi (A^j x - k) \qquad k&\in \mathbb{Z}^2,\, j \in \mathbb{Z},\cr
A&=\bigl(\textstyle{\phantom{-}0 \atop -1}{1 \atop 0}\bigr)
}
\]
ne peuvent jamais avoir de \( \psi \in C^1 \) (parce que
\[
\frac{\partial}{\partial \xi_1} m_0 (\xi_1, \xi_2)|_{\xi_1 = \pi=\xi_2} = 0 = \frac{\partial}{\partial \xi_2} m_0 (\xi_1,\xi_2)|_{\xi_1=\pi=\xi_2}
\]
mène à \[ m_0 (\xi_1,\xi_2) = (1 + e^{i\xi_1})(1+e^{i\xi_2})
\mathcal{F} (\xi_1, \xi_2), \]
ce qui rend
\[ |m_0 (\xi_1, \xi_2)|^2 + |m_0 (\xi_1 + \pi, \xi_2 + \pi)|^2 = 1 \]
impossible).
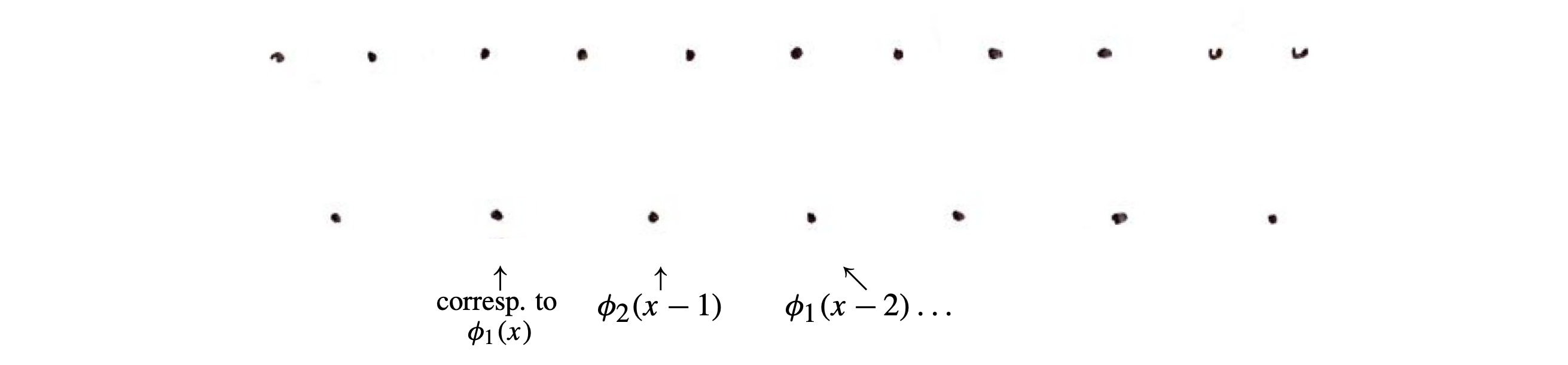
Je n’ai pas la moindre idée de comment imposer de la régularité.
• J’ai une méthode pour trouver des bases biorthogonales symétriques, proches de bases orthonormales. Elle utilise comme point de départ les ondelettes de Battle–Lemarié, et tronque \( m_0 \) pour avoir des supports compacts. Je suis en train de l’incorporer à l’article avec A. Cohen et J. C. Feauveau (toujours pas entièrement rédigé, à ma grande honte! Mais c’est le 1er point sur mon agenda maintenant que le cours est sous contrôle), et je t’enverrai, ainsi qu’à Albert, des détails plus tard.
Voilà tout pour cette lettre!
Un grand bonjour à tous,
Ingrid